Advertisements
Advertisements
प्रश्न
Find the area of a rhombus whose perimeter is 80 m and one of whose diagonal is 24 m.
उत्तर
Given that,
Perimeter of rhombus = 80m
Perimeter of rhombus = 4 × side

⇒ 4a = 80
⇒ a = 20m
Let AC = 24 m
∴ OA =`1/2AC=1/2xx24=12m`
In ΔAOB
`OB^2=AB^2-OA^2`
`⇒OB=sqrt(20^2-12^2)`
`sqrt(400-144)`
`sqrt(256)=16m`
Also BO = OD
[Diagonal of rhombus bisect each other at 90°]
∴ BD = 20B = 2 ×16 = 32 m
∴Area of rhombus = `1/2`×32×24=`384𝑚^2`
[∵Area of rhombus = 12×𝐵𝐷×𝐴𝐶]
APPEARS IN
संबंधित प्रश्न
A park, in the shape of a quadrilateral ABCD, has ∠C = 90°, AB = 9 m, BC = 12 m, CD = 5 m and AD = 8 m. How much area does it occupy?
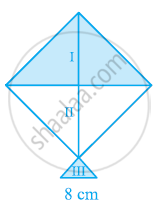
A kite in the shape of a square with a diagonal 32 cm and an isosceles triangles of base 8 cm and sides 6 cm each is to be made of three different shades as shown in the given figure. How much paper of each shade has been used in it?
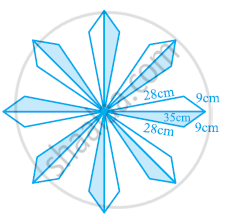
A floral design on a floor is made up of 16 tiles which are triangular, the sides of the triangle being 9 cm, 28 cm and 35 cm (see the given figure). Find the cost of polishing the tiles at the rate of 50p per cm2.
Let Δ be the area of a triangle. Find the area of a triangle whose each side is twice the side of the given triangle.
If each side of a triangle is doubled, the find percentage increase in its area.
Mark the correct alternative in each of the following:
The sides of a triangle are 16 cm, 30 cm, 34 cm. Its area is
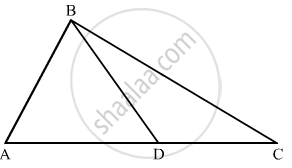
In the given figure, the ratio AD to DC is 3 to 2. If the area of Δ ABC is 40 cm2, what is the area of Δ BDC?
If the length of a median of an equilateral triangle is x cm, then its area is
The adjacent sides of a parallelogram measures 34 m, 20 m and the measure of the diagonal is 42 m. Find the area of parallelogram
The area of a regular hexagon of side ‘a’ is the sum of the areas of the five equilateral triangles with side a.
