Advertisements
Advertisements
प्रश्न
Find the area of a flat circular ring formed by two concentric circles (circles with the same centre) whose radii are 9 cm and 5 cm.
उत्तर
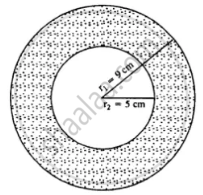
External radius, r1 = 9 cm
Internal radius, r2 = 5 cm
Area of ring = `pir_1^2 - pir_2^2`
= `pi(r_1^2 - r_2^2)`
= `pi(9^(2) - 5^(2))`
= `22/7[81 - 25]`
= `22/7 xx 56`
= 176 cm2
APPEARS IN
संबंधित प्रश्न
In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find the area of the sector formed by the arc. (Use π = `22/7`)
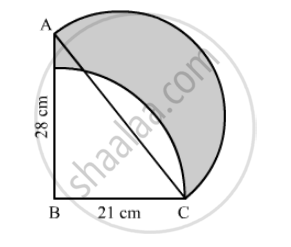
In the following figure, ABC is a right-angled triangle, ∠B = 90°, AB = 28 cm and BC = 21 cm. With AC as diameter a semicircle is drawn and with BC as radius a quarter circle is drawn. Find the area of the shaded region correct to two decimal places.
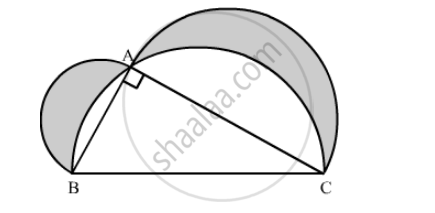
In the following figure, ABC is a right angled triangle in which ∠A = 90°, AB = 21 cm and AC = 28 cm. Semi-circles are described on AB, BC and AC as diameters. Find the area of the shaded region.
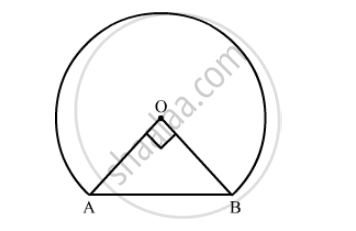
In the following figure, shows the cross-section of railway tunnel. The radius OA of the circular part is 2 m. If ∠AOB = 90°, calculate:
the area of the cross-section.
The circumference of a circle is 100 m. The side of a square inscribed in the circle is
If the radius of a circle is diminished by 10%, then its area is diminished by
The minute hand of a clock is 15 cm long. Calculate the area swept by it in 20 minutes.
The area of a sector of a circle with radius r, making an angle of x° at the centre is
Two circles touch each other externally. The sum of their areas is 58π cm2 and the distance between their centers is 10 cm. Find the radii of the two circles.
Area of circle of radius ‘n’ units is
