Advertisements
Advertisements
प्रश्न
The minute hand of a clock is 15 cm long. Calculate the area swept by it in 20 minutes.
उत्तर
Angle inscribed by the minute hand in 60 minutes = 360°
Angle inscribed by the miniute hand in 20 minutes`=360/60xx20=120°`
We have :
θ = 120 and r = 15 cm
∴ Required area swept by the miniute hand in 20 miniutes = Area of the sector with r = 15 cm and θ = 120°
`= (pi"r"^2theta)/360`
`=3.14xx15xx15xx120/360`
= 235.5 cm2
APPEARS IN
संबंधित प्रश्न
A rectangular park 358 m long and 18 m wide is to be covered with grass, leaving 2.5 m uncovered all around it. Find the area to be laid with grass.
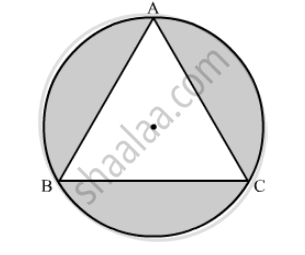
In the following figure, an equilateral triangle ABC of side 6 cm has been inscribed in a circle. Find the area of the shaded region. (Take π = 3.14).
How many revolutions a circular wheel of radius r metres makes in covering a distance of s metres?
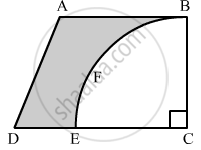
From a thin metallic piece in the shape of a trapezium ABCD in which AB || CD and ∠BCD = 90°, a quarter circle BFEC is removed. Given, AB = BC = 3.5 cm and DE = 2 cm, calculate the area of remaining (shaded) part of metal sheet.
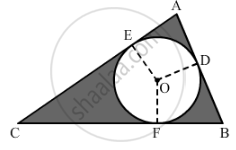
In the given figure, ∆ABC is right-angled at A. Find the area of the shaded region if AB = 6 cm, BC = 10 cm and O is the centre of the incircle of ∆ABC.
Diameter of a circular garden is 9.8 m. Find its area.
Calculate the area of the shotput circle whose radius is 2.135 m.
In figure, a square of diagonal 8 cm is inscribed in a circle. Find the area of the shaded region.
If the radii of two circles are in the ratio of 4 : 3, then their areas are in the ratio of ______.
If the radius of a circle is tripled, the area becomes ______.
