Advertisements
Advertisements
प्रश्न
Find the equations of a line passing through the point (2, 3) and having the x – interecpt of 4 units.
उत्तर
Since x-intercept is 4 units coordinates of point are (4, 0). Equation of a line passing through (2, 3) and (4, 0) is
y - y1 = `(y_2 - y_1)/(x_2 - x_1)(x - x_1)`
⇒ y - 3 = `(0 - 3)/(4 - 2)(x - 2)`
⇒ y - 3 = `(-3)/(2)(x - 2)`
⇒ 2y - 6 = -3x + 6
⇒ 3x + 2y = 12.
APPEARS IN
संबंधित प्रश्न
A(-1, 3), B(4, 2) and C(3, -2) are the vertices of a triangle.
1) Find the coordinates of the centroid G of the triangle
2) Find the equation of the line through G and parallel to AC
State, true or false :
The point (–3, 0) lies on the line x + 3 = 0
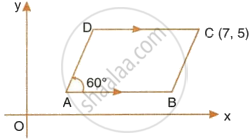
The following figure shows a parallelogram ABCD whose side AB is parallel to the x-axis, ∠A = 60° and vertex C = (7, 5). Find the equations of BC and CD.
Find the equation of the line, whose x-intercept = 5 and y-intercept = 3
Determine whether the line through points (–2, 3) and (4, 1) is perpendicular to the line 3x = y + 1.
Does line 3x = y + 1 bisect the line segment joining the two given points?
The line 4x + 3y = 11 bisects the join of ( 6,m) and (p,9). Find the value of m.
Find the inclination of a line whose gradient is 5.6713
Find the equation of a line whose slope and y-intercept are m = -3, c = -1
Find the equation of a straight line which cuts an intercept of 5 units on Y-axis and is parallel to the line joining the points (3, – 2) and (1, 4).
Find the equation of a line passing through (3, – 2) and perpendicular to the line.
x - 3y + 5 = 0.
