Advertisements
Advertisements
प्रश्न
Find the equations of a line passing through the point (2, 3) and having the x – interecpt of 4 units.
उत्तर
Since x-intercept is 4 units coordinates of point are (4, 0). Equation of a line passing through (2, 3) and (4, 0) is
y - y1 = `(y_2 - y_1)/(x_2 - x_1)(x - x_1)`
⇒ y - 3 = `(0 - 3)/(4 - 2)(x - 2)`
⇒ y - 3 = `(-3)/(2)(x - 2)`
⇒ 2y - 6 = -3x + 6
⇒ 3x + 2y = 12.
APPEARS IN
संबंधित प्रश्न
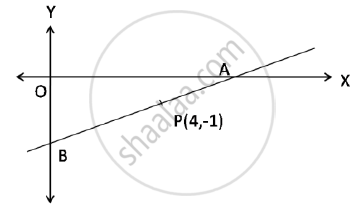
A line AB meets X – axis at A and Y –axis at B. P (4, -1) divides AB in the ratio 1 : 2.
1) Find the coordinates of A and B.
2) Find the equation of the line through P and perpendicular to AB.
A(2, 5), B(–1, 2) and C(5, 8) are the vertices of a triangle ABC, `M' is a point on AB such that AM: MB = 1: 2. Find the coordinates of 'M'. Hence find the equation of the line passing through the points C and M
Find, which of the following points lie on the line x – 2y + 5 = 0 :
(1, 3)
The line x – 6y + 11 = 0 bisects the join of (8, −1) and (0, k). Find the value of k.
The point P divides the join of (2, 1) and (−3, 6) in the ratio 2 : 3. Does P lies on the line x − 5y + 15 = 0?
Find the equation of the line whose slope is `-5/6` and x-intercept is 6.
A(1, 4), B(3, 2) and C(7, 5) are vertices of a triangle ABC. Find the equation of a line, through the centroid and parallel to AB.
The line segment formed by the points (3, 7) and (-7, z) is bisected by the line 3x + 4y =18. Find the value of z.
Find the inclination of a line whose gradient is 5.6713
Find the inclination of a line whose gradient is 0.5317
