Advertisements
Advertisements
प्रश्न
Find the volume of a cone whose slant height is 17 cm and radius of base is 8 cm.
उत्तर
Slant height (l) = 17 cm
Radius (r) = 8 cm
But, l2 = r2 + h2
`=>` h2 = 12 – r2
`=>` h2 = 172 – 82
`=>` h2 = 289 – 64 = 225 = (15)2
∴ h = 15
Now, volume of cone = `1/3pir^2h`
= `1/3 xx 22/7 xx 8 xx 8 xx 15 cm^3`
= `7040/7cm^3`
= 1005.71 cm3
APPEARS IN
संबंधित प्रश्न
Find the curved surface area of a cone, if its slant height is 60 cm and the radius of its base is 21 cm.
The radius of a cone is 5 cm and vertical height is 12 cm. Find the area of the curved surface.
The radius of a cone is 7 cm and area of curved surface is 176 `cm^2`. Find the slant height.
Curved surface area of a cone is 308 cm2 and its slant height is 14 cm. Find the radius of the base and total surface area of the cone.
The radius and height of a right circular cone are in the ratio 5 : 12 and its volume is 2512 cubic cm. Find the slant height and radius of the cone. (Use it 𝜋 = 3.14).
A right angled triangle of which the sides containing he right angle are 6.3 cm and lo cm in length, is made to turn round on the longer side. Find the volume of the solid, thus generated. Also, find its curved surface area.
Find what length of canvas, 1.5 m in width, is required to make a conical tent 48 m in diameter and 7 m in height. Given that 10% of the canvas is used in folds and stitchings. Also, find the cost of the canvas at the rate of Rs. 24 per metre.
A solid cone of height 8 cm and base radius 6 cm is melted and recast into identical cones, each of height 2 cm and diameter 1 cm. Find the number of cones formed.
The volume of a conical tent is 1232 m3 and the area of the base floor is 154 m2. Calculate the:
- radius of the floor,
- height of the tent,
- length of the canvas required to cover this conical tent if its width is 2 m.
A hemispherical bowl of diameter 7.2 cm is filled completely with chocolate sauce. This sauce is poured into an inverted cone of radius 4.8 cm. Find the height of the cone if it is completely filled.
A cone of height 15 cm and diameter 7 cm is mounted on a hemisphere of same diameter. Determine the volume of the solid thus formed.
Curved surface area of a cone is 251.2 cm2 and radius of its base is 8 cm. Find its slant height and perpendicular height. (π = 3.14)
What will be the cost of making a closed cone of tin sheet having radius of base 6 m and slant height 8 m if the rate of making is Rs.10 per sq.m? `(π = 22/7)`
The curved surface area of a right circular cone of radius 11.3 cm is 710 cm2. What is the slant height of the cone ?
A buoy is made in the form of a hemisphere surmounted by a right circular cone whose circular base coincides with the plane surface of the hemisphere. The radius of the base of the cone is 3.5 m and its volume is two-third the volume of hemisphere. Calculate the height of the cone and the surface area of the buoy, correct to two decimal places.
Find the cost of painting a hemispherical dome of diameter 10 m at the rate of Rs 1.40 per square metre.
The volume of a conical tent is 1232 m3 and the area of the base floor is 154 m2. Calculate the: height of the tent.
The given figure shows the cross-section of a cone, a cylinder and a hemisphere all with the same diameter 10 cm and the other dimensions are as shown. Calculate: the total volume of the solid.
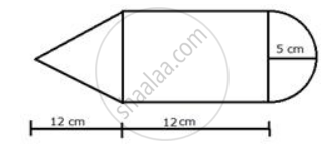
The ratio of the base area and the curved surface of a conical tent is 40: 41. If the height is 18 m, Find the air capacity of the tent in terms of n.
