Advertisements
Advertisements
प्रश्न
Find, which of the following points lie on the line x – 2y + 5 = 0 :
(5, 5)
उत्तर
The given line is x − 2y + 5 = 0.
Substituting x = 5 and y = 5 in the given equation, we have:
L.H.S. = 5 − 2 × 5 + 5
= 5 − 10 + 5
= 10 − 10
= 0 = R.H.S.
Thus, the point (5, 5) lies on the given line.
APPEARS IN
संबंधित प्रश्न
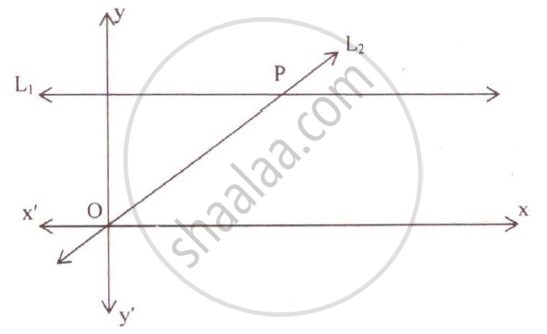
Given the equation of line L, is y = 4.
(1) Write the slope of line L2, if L2, is the bisector of angle O.
(2) Write the co–ordinates of point P.
(3) Find the equation of L2.
Find, which of the following points lie on the line x – 2y + 5 = 0 :
(0, 5)
State, true or false :
If the point (2, a) lies on the line 2x – y = 3, then a = 5.
Show that the lines 2x + 5y = 1, x – 3y = 6 and x + 5y + 2 = 0 are concurrent.
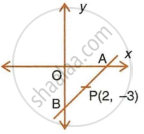
A and B are two points on the x-axis and y-axis respectively. P(2, −3) is the mid point of AB. Find the
- co-ordinates of A and B
- slope of line AB
- equation of line AB.
The equation of a line is 3x + 4y – 7 = 0. Find:
- the slope of the line.
- the equation of a line perpendicular to the given line and passing through the intersection of the lines x – y + 2 = 0 and 3x + y – 10 = 0.
The line y = 6- `(3"x")/2` passes through the point (r,3). Find the value of r.
Find the equation of a line whose slope and y-intercept are m = -3, c = -1
X(4,9), Y(-5,4) and Z(7,-4) are the vertices of a triangle. Find the equation of the altitude of the triangle through X.
PQ is straight line of 13 units. If P has coordinate (2, 5) and Q has coordinate (x, – 7) find the possible values of x.
