Advertisements
Advertisements
प्रश्न
Find the zeroes of the polynomial `x^2 + x – p(p + 1) `
उत्तर
f(x) = x2 + x – p (p + 1)
By adding and subtracting px, we get
`f(x) = x^2 + px + x – px – p(p + 1)`
= `x^2 + (p + 1) x – px –p (p + 1)`
=` x[x + (p + 1)] –p [x + (p + 1)]`
= `[x + (p + 1)] (x – p)`
`f(x) = 0`
⇒`[x + (p + 1)] (x – p) = 0`
⇒ `[x + (p + 1)] = 0 or (x – p) = 0`
⇒ `x = – (p + 1) or x = p`
So, the zeroes of f(x) are – (p + 1) and p.
APPEARS IN
संबंधित प्रश्न
If 1is a zero of the quadratic polynomial `ax^2 – 3(a – 1)x – 1`is 1, then find the value of a.
Write the zeros of the polynomial `f(x) = x^2 – x – 6`.
Find the zeroes of the quadratic polynomial `f(x) = 6x^2 – 3.`
If one of the zeroes of a quadratic polynomial of the form x² + ax + b is the negative of the other, then it ______.
If one of the zeroes of the cubic polynomial x3 + px² + qx + r is -1, then the product of the other two zeroes is ______.
If p(x) is a polynomial of at least degree one and p(k) = 0, then k is known as ______.
A polynomial of degree n has ______.
If 4x² – 6x – m is divisible by x – 3, the value of m is exact divisor of ______.
The number of polynomials having zeroes as -2 and 5 is ______.
The graph of y = f(x) is shown in the figure for some polynomial f(x).
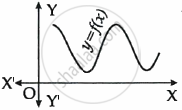
The number of zeroes of f(x) is ______.
