Advertisements
Advertisements
प्रश्न
Four balls are to be drawn without replacement from a box containing 8 red and 4 white balls. If X denotes the number of red balls drawn, then find the probability distribution of X.
उत्तर
As, four balls are to be drawn without replacement and X denotes the number of red balls drawn
So, X is a random variable that can take values 0, 1, 2, 3 or 4
Now,
\[P\left( X = 0 \right) = P\left( \text{ All white balls } \right) = P\left( WWWW \right) = \frac{4}{12} \times \frac{3}{11} \times \frac{2}{10} \times \frac{1}{9} = \frac{1}{495}, \]
\[P\left( X = 1 \right) = P\left( \text{ One red balls and three white balls } \right) = P\left( RWWW \right) + P\left( WRWW \right) + P\left( WWRW \right) + P\left( WWWR \right)\]
\[ = \frac{8}{12} \times \frac{4}{11} \times \frac{3}{10} \times \frac{2}{9} + \frac{4}{12} \times \frac{8}{11} \times \frac{3}{10} \times \frac{2}{9} + \frac{4}{12} \times \frac{3}{11} \times \frac{8}{10} \times \frac{2}{9} + \frac{4}{12} \times \frac{3}{11} \times \frac{2}{10} \times \frac{8}{9}\]
\[ = 4 \times \frac{8}{495} = \frac{32}{495}, \]
\[P\left( X = 2 \right) = P\left( \text{ Two red balls and two white balls } \right) = P\left( RRWW \right) + P\left( RWRW \right) + P\left( RWWR \right) + P\left( WWRR \right) + P\left( WRWR \right) + P\left( WRRW \right)\]
\[ = \frac{8}{12} \times \frac{7}{11} \times \frac{4}{10} \times \frac{3}{9} + \frac{8}{12} \times \frac{4}{11} \times \frac{7}{10} \times \frac{3}{9} + \frac{8}{12} \times \frac{4}{11} \times \frac{3}{10} \times \frac{7}{9} + \frac{4}{12} \times \frac{3}{11} \times \frac{8}{10} \times \frac{7}{9} + \frac{4}{12} \times \frac{8}{11} \times \frac{3}{10} \times \frac{7}{9} + \frac{4}{12} \times \frac{8}{11} \times \frac{7}{10} \times \frac{3}{9}\]
\[ = 6 \times \frac{28}{495} = \frac{168}{495}, \]
\[P\left( X = 3 \right) = P\left( \text{ Three red balls and one white ball } \right) = P\left( RRRW \right) + P\left( RRWR \right) + P\left( RWRR \right) + P\left( WRRR \right)\]
\[ = \frac{8}{12} \times \frac{7}{11} \times \frac{6}{10} \times \frac{4}{9} + \frac{8}{12} \times \frac{7}{11} \times \frac{4}{10} \times \frac{6}{9} + \frac{8}{12} \times \frac{4}{11} \times \frac{7}{10} \times \frac{6}{9} + \frac{4}{12} \times \frac{8}{11} \times \frac{7}{10} \times \frac{6}{9}\]
\[ = 4 \times \frac{56}{495} = \frac{224}{495}, \]
\[P\left( X = 4 \right) = P\left( \text{ All red balls } \right) = P\left( RRRR \right) = \frac{8}{12} \times \frac{7}{11} \times \frac{6}{10} \times \frac{5}{9}\]
\[ = \frac{70}{495}\]
So, the probability distribution of X is as follows:
| x | 0 | 1 | 2 | 3 | 4 |
| P(X) | \[\frac{1}{495}\] |
\[\frac{32}{495}\]
|
\[\frac{168}{495}\]
|
\[\frac{224}{495}\]
|
\[\frac{70}{495}\]
|
APPEARS IN
संबंधित प्रश्न
From a lot of 15 bulbs which include 5 defectives, a sample of 4 bulbs is drawn one by one with replacement. Find the probability distribution of number of defective bulbs. Hence find the mean of the distribution.
State the following are not the probability distributions of a random variable. Give reasons for your answer.
| X | 0 | 1 | 2 | 3 | 4 |
| P(X) | 0.1 | 0.5 | 0.2 | -0.1 | 0.3 |
State the following are not the probability distributions of a random variable. Give reasons for your answer.
| Z | 3 | 2 | 1 | 0 | -1 |
| P(Z) | 0.3 | 0.2 | 0.4 | 0.1 | 0.05 |
Find the probability distribution of number of heads in four tosses of a coin.
A random variable X has the following probability distribution.
| X | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
| P(X) | 0 | k | 2k | 2k | 3k | k2 |
2k2 |
7k2 + k |
Determine
(i) k
(ii) P (X < 3)
(iii) P (X > 6)
(iv) P (0 < X < 3)
Four cards are drawn simultaneously from a well shuffled pack of 52 playing cards. Find the probability distribution of the number of aces.
Five defective bolts are accidently mixed with twenty good ones. If four bolts are drawn at random from this lot, find the probability distribution of the number of defective bolts.
Two cards are drawn successively without replacement from a well shuffled pack of 52 cards. Find the probability distribution of the number of aces.
Three cards are drawn successively with replacement from a well-shuffled deck of 52 cards. A random variable X denotes the number of hearts in the three cards drawn. Determine the probability distribution of X.
Find the mean and standard deviation of each of the following probability distribution :
| xi : | 1 | 2 | 3 | 4 |
| pi : | 0.4 | 0.3 | 0.2 | 0.1 |
Find the mean and standard deviation of each of the following probability distribution :
| xi : | -3 | -1 | 0 | 1 | 3 |
| pi : | 0.05 | 0.45 | 0.20 | 0.25 | 0.05 |
Two cards are selected at random from a box which contains five cards numbered 1, 1, 2, 2, and 3. Let X denote the sum and Y the maximum of the two numbers drawn. Find the probability distribution, mean and variance of X and Y.
A box contains 13 bulbs, out of which 5 are defective. 3 bulbs are randomly drawn, one by one without replacement, from the box. Find the probability distribution of the number of defective bulbs.
In a game, a man wins Rs 5 for getting a number greater than 4 and loses Rs 1 otherwise, when a fair die is thrown. The man decided to thrown a die thrice but to quit as and when he gets a number greater than 4. Find the expected value of the amount he wins/loses.
If X denotes the number on the upper face of a cubical die when it is thrown, find the mean of X.
Using the truth table verify that p ∨ (q ∧ r) ≡ (p ∨ q) ∧ (p ∨ r).
If the demand function is D = 150 - p2 - 3p, find marginal revenue, average revenue and elasticity of demand for price p = 3.
A departmental store gives trafnfng to the salesmen in service followed by a test. It is experienced that the performance regarding sales of any salesman is linearly related to the scores secured by him. The following data gives the test scores and sales made by nine (9) salesmen during a fixed period.
| Test scores (X) | 16 | 22 | 28 | 24 | 29 | 25 | 16 | 23 | 24 |
| Sales (Y) (₹ in hundreds) | 35 | 42 | 57 | 40 | 54 | 51 | 34 | 47 | 45 |
(a) Obtain the line of regression of Y on X.
(b) Estimate Y when X = 17.
Three different aeroplanes are to be assigned to carry three cargo consignments with a view to maximize profit. The profit matrix (in lakhs of ₹) is as follows :
| Aeroplanes | Cargo consignments | ||
| C1 | C2 | C3 | |
| A1 | 1 | 4 | 5 |
| A2 | 2 | 3 | 3 |
| A3 | 3 | 1 | 2 |
How should the cargo consignments be assigned to the aeroplanes to maximize the profit?
A random variable X has the following probability distribution :
| X = x | -2 | -1 | 0 | 1 | 2 | 3 |
| P(x) | 0.1 | k | 0.2 | 2k | 0.3 | k |
Find the value of k and calculate mean.
A die is thrown 4 times. If ‘getting an odd number’ is a success, find the probability of 2 successes
The probability that a bulb produced by a factory will fuse after 200 days of use is 0.2. Let X denote the number of bulbs (out of 5) that fuse after 200 days of use. Find the probability of X = 0
The probability that a bulb produced by a factory will fuse after 200 days of use is 0.2. Let X denote the number of bulbs (out of 5) that fuse after 200 days of use. Find the probability of X ≤ 1
10 balls are marked with digits 0 to 9. If four balls are selected with replacement. What is the probability that none is marked 0?
Solve the following problem :
Following is the probability distribution of a r.v.X.
| X | – 3 | – 2 | –1 | 0 | 1 | 2 | 3 |
| P(X = x) | 0.05 | 0.1 | 0.15 | 0.20 | 0.25 | 0.15 | 0.1 |
Find the probability that X is positive.
Solve the following problem :
Following is the probability distribution of a r.v.X.
| x | – 3 | – 2 | –1 | 0 | 1 | 2 | 3 |
| P(X = x) | 0.05 | 0.1 | 0.15 | 0.20 | 0.25 | 0.15 | 0.1 |
Find the probability that X is non-negative
Solve the following problem :
Find the probability of the number of successes in two tosses of a die, where success is defined as number greater than 4.
Solve the following problem :
The probability that a machine will produce all bolts in a production run within the specification is 0.9. A sample of 3 machines is taken at random. Calculate the probability that all machines will produce all bolts in a production run within the specification.
Solve the following problem :
It is observed that it rains on 10 days out of 30 days. Find the probability that it rains on exactly 3 days of a week.
Four balls are to be drawn without replacement from a box containing 8 red and 4 white balls. If X denotes the number of red ball drawn, find the probability distribution of X.
Two biased dice are thrown together. For the first die P(6) = `1/2`, the other scores being equally likely while for the second die, P(1) = `2/5` and the other scores are equally likely. Find the probability distribution of ‘the number of ones seen’.
Find the probability distribution of the number of successes in two toves of a die where a success is define as:- Six appeared on at least one die.
If the p.m.f of a r. v. X is
P(x) = `c/x^3`, for x = 1, 2, 3
= 0, otherwise
then E(X) = ______.
Two balls are drawn at random one by one with replacement from an urn containing equal number of red balls and green balls. Find the probability distribution of number of red balls. Also, find the mean of the random variable.
The probability that a bomb will hit the target is 0.8. Complete the following activity to find, the probability that, out of 5 bombs exactly 2 will miss the target.
Solution: Here, n = 5, X =number of bombs that hit the target
p = probability that bomb will hit the target = `square`
∴ q = 1 - p = `square`
Here, `X∼B(5,4/5)`
∴ P(X = x) = `""^"n""C"_x"P"^x"q"^("n" - x) = square`
P[Exactly 2 bombs will miss the target] = P[Exactly 3 bombs will hit the target]
= P(X = 3)
=`""^5"C"_3(4/5)^3(1/5)^2=10(4/5)^3(1/5)^2`
∴ P(X = 3) = `square`
Kiran plays a game of throwing a fair die 3 times but to quit as and when she gets a six. Kiran gets +1 point for a six and –1 for any other number.
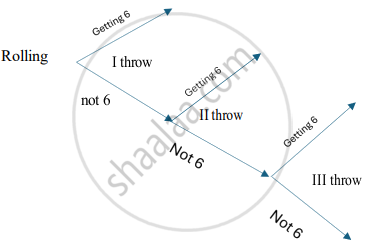
- If X denotes the random variable “points earned” then what are the possible values X can take?
- Find the probability distribution of this random variable X.
- Find the expected value of the points she gets.
