Advertisements
Advertisements
प्रश्न
Four balls are to be drawn without replacement from a box containing 8 red and 4 white balls. If X denotes the number of red balls drawn, then find the probability distribution of X.
उत्तर
As, four balls are to be drawn without replacement and X denotes the number of red balls drawn
So, X is a random variable that can take values 0, 1, 2, 3 or 4
Now,
\[P\left( X = 0 \right) = P\left( \text{ All white balls } \right) = P\left( WWWW \right) = \frac{4}{12} \times \frac{3}{11} \times \frac{2}{10} \times \frac{1}{9} = \frac{1}{495}, \]
\[P\left( X = 1 \right) = P\left( \text{ One red balls and three white balls } \right) = P\left( RWWW \right) + P\left( WRWW \right) + P\left( WWRW \right) + P\left( WWWR \right)\]
\[ = \frac{8}{12} \times \frac{4}{11} \times \frac{3}{10} \times \frac{2}{9} + \frac{4}{12} \times \frac{8}{11} \times \frac{3}{10} \times \frac{2}{9} + \frac{4}{12} \times \frac{3}{11} \times \frac{8}{10} \times \frac{2}{9} + \frac{4}{12} \times \frac{3}{11} \times \frac{2}{10} \times \frac{8}{9}\]
\[ = 4 \times \frac{8}{495} = \frac{32}{495}, \]
\[P\left( X = 2 \right) = P\left( \text{ Two red balls and two white balls } \right) = P\left( RRWW \right) + P\left( RWRW \right) + P\left( RWWR \right) + P\left( WWRR \right) + P\left( WRWR \right) + P\left( WRRW \right)\]
\[ = \frac{8}{12} \times \frac{7}{11} \times \frac{4}{10} \times \frac{3}{9} + \frac{8}{12} \times \frac{4}{11} \times \frac{7}{10} \times \frac{3}{9} + \frac{8}{12} \times \frac{4}{11} \times \frac{3}{10} \times \frac{7}{9} + \frac{4}{12} \times \frac{3}{11} \times \frac{8}{10} \times \frac{7}{9} + \frac{4}{12} \times \frac{8}{11} \times \frac{3}{10} \times \frac{7}{9} + \frac{4}{12} \times \frac{8}{11} \times \frac{7}{10} \times \frac{3}{9}\]
\[ = 6 \times \frac{28}{495} = \frac{168}{495}, \]
\[P\left( X = 3 \right) = P\left( \text{ Three red balls and one white ball } \right) = P\left( RRRW \right) + P\left( RRWR \right) + P\left( RWRR \right) + P\left( WRRR \right)\]
\[ = \frac{8}{12} \times \frac{7}{11} \times \frac{6}{10} \times \frac{4}{9} + \frac{8}{12} \times \frac{7}{11} \times \frac{4}{10} \times \frac{6}{9} + \frac{8}{12} \times \frac{4}{11} \times \frac{7}{10} \times \frac{6}{9} + \frac{4}{12} \times \frac{8}{11} \times \frac{7}{10} \times \frac{6}{9}\]
\[ = 4 \times \frac{56}{495} = \frac{224}{495}, \]
\[P\left( X = 4 \right) = P\left( \text{ All red balls } \right) = P\left( RRRR \right) = \frac{8}{12} \times \frac{7}{11} \times \frac{6}{10} \times \frac{5}{9}\]
\[ = \frac{70}{495}\]
So, the probability distribution of X is as follows:
| x | 0 | 1 | 2 | 3 | 4 |
| P(X) | \[\frac{1}{495}\] |
\[\frac{32}{495}\]
|
\[\frac{168}{495}\]
|
\[\frac{224}{495}\]
|
\[\frac{70}{495}\]
|
APPEARS IN
संबंधित प्रश्न
Suppose that two cards are drawn at random from a deck of cards. Let X be the number of aces obtained. Then the value of E(X) is
(A) `37/221`
(B) 5/13
(C) 1/13
(D) 2/13
If the probability that a fluorescent light has a useful life of at least 800 hours is 0.9, find the probabilities that among 20 such lights at least 2 will not have a useful life of at least 800 hours. [Given : (0⋅9)19 = 0⋅1348]
Three persons A, B and C shoot to hit a target. If A hits the target four times in five trials, B hits it three times in four trials and C hits it two times in three trials, find the probability that:
1) Exactly two persons hit the target.
2) At least two persons hit the target.
3) None hit the target.
Two numbers are selected at random (without replacement) from positive integers 2, 3, 4, 5, 6 and 7. Let X denote the larger of the two numbers obtained. Find the mean and variance of the probability distribution of X.
A random variable X has the following probability distribution:
| Values of X : | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| P (X) : | a | 3a | 5a | 7a | 9a | 11a | 13a | 15a | 17a |
Determine:
(i) The value of a
(ii) P (X < 3), P (X ≥ 3), P (0 < X < 5).
Two cards are drawn successively with replacement from well shuffled pack of 52 cards. Find the probability distribution of the number of aces.
Two cards are drawn successively with replacement from a well shuffled pack of 52 cards. Find the probability distribution of the number of kings.
Find the mean and standard deviation of each of the following probability distribution:
| xi : | −1 | 0 | 1 | 2 | 3 |
| pi : | 0.3 | 0.1 | 0.1 | 0.3 | 0.2 |
Find the mean and standard deviation of each of the following probability distribution :
| xi : | -3 | -1 | 0 | 1 | 3 |
| pi : | 0.05 | 0.45 | 0.20 | 0.25 | 0.05 |
A box contains 13 bulbs, out of which 5 are defective. 3 bulbs are randomly drawn, one by one without replacement, from the box. Find the probability distribution of the number of defective bulbs.
An urn contains 5 red and 2 black balls. Two balls are randomly drawn, without replacement. Let X represent the number of black balls drawn. What are the possible values of X ? Is X a random variable ? If yes, then find the mean and variance of X.
Write the values of 'a' for which the following distribution of probabilities becomes a probability distribution:
| X= xi: | -2 | -1 | 0 | 1 |
| P(X= xi) : |
\[\frac{1 - a}{4}\]
|
\[\frac{1 + 2a}{4}\]
|
\[\frac{1 - 2a}{4}\]
|
\[\frac{1 + a}{4}\]
|
If X denotes the number on the upper face of a cubical die when it is thrown, find the mean of X.
If the probability distribution of a random variable X is as given below:
Write the value of P (X ≤ 2).
| X = xi : | 1 | 2 | 3 | 4 |
| P (X = xi) : | c | 2c | 4c | 4c |
A random variable has the following probability distribution:
| X = xi : | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
| P (X = xi) : | 0 | 2 p | 2 p | 3 p | p2 | 2 p2 | 7 p2 | 2 p |
The value of p is
Using the truth table verify that p ∨ (q ∧ r) ≡ (p ∨ q) ∧ (p ∨ r).
If random variable X has probability distribution function.
f(x) = `c/x`, 1 < x < 3, c > 0, find c, E(x) and Var(X)
Verify whether the following function can be regarded as probability mass function (p.m.f.) for the given values of X :
| X | -1 | 0 | 1 |
| P(X = x) | -0.2 | 1 | 0.2 |
The p.m.f. of a random variable X is
`"P"(x) = 1/5` , for x = I, 2, 3, 4, 5
= 0 , otherwise.
Find E(X).
The defects on a plywood sheet occur at random with an average of the defect per 50 sq. ft. What Is the probability that such sheet will have-
(a) No defects
(b) At least one defect
[Use e-1 = 0.3678]
From the following data, find the crude death rates (C.D.R.) for Town I and Town II, and comment on the results :
| Age Group (in years) | Town I | Town II | ||
| Population | No. of deaths | Population | No. of deaths | |
| 0-10 | 1500 | 45 | 6000 | 150 |
| 10-25 | 5000 | 30 | 6000 | 40 |
| 25 - 45 | 3000 | 15 | 5000 | 20 |
| 45 & above | 500 | 22 | 3000 | 54 |
A random variable X has the following probability distribution :
| x = x | 0 | 1 | 2 | 3 | 7 | |||
| P(X=x) | 0 | k | 2k | 2k | 3k | k2 | 2k2 | 7k2 + k |
Determine (i) k
(ii) P(X> 6)
(iii) P(0<X<3).
Determine whether each of the following is a probability distribution. Give reasons for your answer.
| y | –1 | 0 | 1 |
| P(y) | 0.6 | 0.1 | 0.2 |
Find the probability distribution of the number of successes in two tosses of a die if success is defined as getting a number greater than 4.
A coin is biased so that the head is 3 times as likely to occur as tail. Find the probability distribution of number of tails in two tosses.
Find the probability of throwing at most 2 sixes in 6 throws of a single die.
Defects on plywood sheet occur at random with the average of one defect per 50 sq.ft. Find the probability that such a sheet has:
- no defect
- at least one defect
Use e−1 = 0.3678
Solve the following problem :
Find the probability of the number of successes in two tosses of a die, where success is defined as six appears in at least one toss.
Solve the following problem :
The probability that a machine will produce all bolts in a production run within the specification is 0.9. A sample of 3 machines is taken at random. Calculate the probability that all machines will produce all bolts in a production run within the specification.
Solve the following problem :
It is observed that it rains on 10 days out of 30 days. Find the probability that it rains on at most 2 days of a week.
A discrete random variable X has the probability distribution given as below:
| X | 0.5 | 1 | 1.5 | 2 |
| P(X) | k | k2 | 2k2 | k |
Find the value of k
A discrete random variable X has the probability distribution given as below:
| X | 0.5 | 1 | 1.5 | 2 |
| P(X) | k | k2 | 2k2 | k |
Determine the mean of the distribution.
Two probability distributions of the discrete random variable X and Y are given below.
| X | 0 | 1 | 2 | 3 |
| P(X) | `1/5` | `2/5` | `1/5` | `1/5` |
| Y | 0 | 1 | 2 | 3 |
| P(Y) | `1/5` | `3/10` | `2/10` | `1/10` |
Prove that E(Y2) = 2E(X).
Find the probability distribution of the maximum of the two scores obtained when a die is thrown twice. Determine also the mean of the distribution.
The random variable X can take only the values 0, 1, 2. Given that P(X = 0) = P(X = 1) = p and that E(X2) = E[X], find the value of p
Let X be a discrete random variable whose probability distribution is defined as follows:
P(X = x) = `{{:("k"(x + 1), "for" x = 1"," 2"," 3"," 4),(2"k"x, "for" x = 5"," 6"," 7),(0, "Otherwise"):}`
where k is a constant. Calculate Standard deviation of X.
The probability distribution of a random variable x is given as under:
P(X = x) = `{{:("k"x^2, "for" x = 1"," 2"," 3),(2"k"x, "for" x = 4"," 5"," 6),(0, "otherwise"):}`
where k is a constant. Calculate P(X ≥ 4)
A random variable x has to following probability distribution.
| X | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
| P(x) | 0 | k | 2k | 2k | 3k | k2 | 2k2 | 7k2 + k |
Determine
Kiran plays a game of throwing a fair die 3 times but to quit as and when she gets a six. Kiran gets +1 point for a six and –1 for any other number.
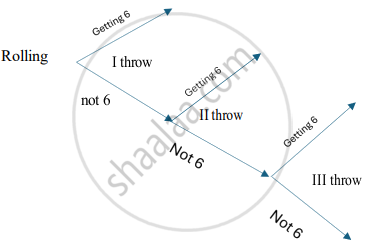
- If X denotes the random variable “points earned” then what are the possible values X can take?
- Find the probability distribution of this random variable X.
- Find the expected value of the points she gets.
