Advertisements
Advertisements
प्रश्न
Kiran plays a game of throwing a fair die 3 times but to quit as and when she gets a six. Kiran gets +1 point for a six and –1 for any other number.
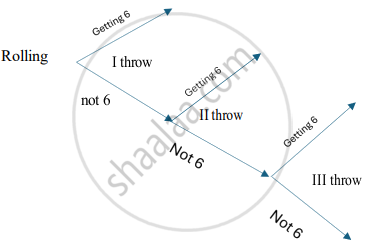
- If X denotes the random variable “points earned” then what are the possible values X can take?
- Find the probability distribution of this random variable X.
- Find the expected value of the points she gets.
उत्तर
i. X = −3, −1, 0, 1
ii. P(X = −3) = `5/6 xx 5/6 xx 5/6 = 125/216`
P(X = −1) = `5/6 xx 5/6 xx 1/6 = 25/216`
P(X = 0) = `5/6 xx 1/6 = 5/36`
P(X = 1) = `1/6`
Required probability distribution:
| X | −3 | −1 | 0 | 1 |
| P(X) | `125/216` | `25/216` | `5/36` | `1/6` |
iii. Expected value = `sumpx`
= `(−3)(125/216) + (-1)(25/216) + 0 + (1/6)`
= `-91/54`
= `-1 37/54 ≈ -1.69`
APPEARS IN
संबंधित प्रश्न
Let, X denote the number of colleges where you will apply after your results and P(X = x) denotes your probability of getting admission in x number of colleges. It is given that
where k is a positive constant. Find the value of k. Also find the probability that you will get admission in (i) exactly one college (ii) at most 2 colleges (iii) at least 2 colleges.
Two numbers are selected at random (without replacement) from positive integers 2, 3, 4, 5, 6 and 7. Let X denote the larger of the two numbers obtained. Find the mean and variance of the probability distribution of X.
Two cards are drawn from a well shuffled pack of 52 cards. Find the probability distribution of the number of aces.
Two dice are thrown together and the number appearing on them noted. X denotes the sum of the two numbers. Assuming that all the 36 outcomes are equally likely, what is the probability distribution of X?
Two cards are drawn successively with replacement from a well shuffled pack of 52 cards. Find the probability distribution of the number of kings.
The probability distribution of a random variable X is given below:
| x | 0 | 1 | 2 | 3 |
| P(X) | k |
\[\frac{k}{2}\]
|
\[\frac{k}{4}\]
|
\[\frac{k}{8}\]
|
Determine the value of k .
Two bad eggs are accidently mixed up with ten good ones. Three eggs are drawn at random with replacement from this lot. Compute the mean for the number of bad eggs drawn.
A die is tossed twice. A 'success' is getting an odd number on a toss. Find the variance of the number of successes.
An urn contains 5 red and 2 black balls. Two balls are randomly drawn, without replacement. Let X represent the number of black balls drawn. What are the possible values of X ? Is X a random variable ? If yes, then find the mean and variance of X.
Write the values of 'a' for which the following distribution of probabilities becomes a probability distribution:
| X= xi: | -2 | -1 | 0 | 1 |
| P(X= xi) : |
\[\frac{1 - a}{4}\]
|
\[\frac{1 + 2a}{4}\]
|
\[\frac{1 - 2a}{4}\]
|
\[\frac{1 + a}{4}\]
|
For what value of k the following distribution is a probability distribution?
| X = xi : | 0 | 1 | 2 | 3 |
| P (X = xi) : | 2k4 | 3k2 − 5k3 | 2k − 3k2 | 3k − 1 |
Find the mean of the following probability distribution:
| X= xi: | 1 | 2 | 3 |
| P(X= xi) : |
\[\frac{1}{4}\]
|
\[\frac{1}{8}\]
|
\[\frac{5}{8}\]
|
A random variable has the following probability distribution:
| X = xi : | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
| P (X = xi) : | 0 | 2 p | 2 p | 3 p | p2 | 2 p2 | 7 p2 | 2 p |
The value of p is
Mark the correct alternative in the following question:
The probability distribution of a discrete random variable X is given below:
| X: | 2 | 3 | 4 | 5 |
| P(X): |
\[\frac{5}{k}\]
|
\[\frac{7}{k}\]
|
\[\frac{9}{k}\]
|
\[\frac{11}{k}\] |
The value of k is .
Two fair coins are tossed simultaneously. If X denotes the number of heads, find the probability distribution of X. Also find E(X).
A departmental store gives trafnfng to the salesmen in service followed by a test. It is experienced that the performance regarding sales of any salesman is linearly related to the scores secured by him. The following data gives the test scores and sales made by nine (9) salesmen during a fixed period.
| Test scores (X) | 16 | 22 | 28 | 24 | 29 | 25 | 16 | 23 | 24 |
| Sales (Y) (₹ in hundreds) | 35 | 42 | 57 | 40 | 54 | 51 | 34 | 47 | 45 |
(a) Obtain the line of regression of Y on X.
(b) Estimate Y when X = 17.
Write the negation of the following statements :
(a) Chetan has black hair and blue eyes.
(b) ∃ x ∈ R such that x2 + 3 > 0.
Verify whether the following function can be regarded as probability mass function (p.m.f.) for the given values of X :
| X | -1 | 0 | 1 |
| P(X = x) | -0.2 | 1 | 0.2 |
Find expected value and variance of X, where X is number obtained on uppermost face when a fair die is thrown.
Solve the following :
Identify the random variable as either discrete or continuous in each of the following. Write down the range of it.
20 white rats are available for an experiment. Twelve rats are male. Scientist randomly selects 5 rats number of female rats selected on a specific day
A pair of dice is thrown 3 times. If getting a doublet is considered a success, find the probability of two successes
The probability that a bulb produced by a factory will fuse after 200 days of use is 0.2. Let X denote the number of bulbs (out of 5) that fuse after 200 days of use. Find the probability of X ≤ 1
In a multiple choice test with three possible answers for each of the five questions, what is the probability of a candidate getting four or more correct answers by random choice?
Solve the following problem :
Following is the probability distribution of a r.v.X.
| x | – 3 | – 2 | –1 | 0 | 1 | 2 | 3 |
| P(X = x) | 0.05 | 0.1 | 0.15 | 0.20 | 0.25 | 0.15 | 0.1 |
Find the probability that X is non-negative
Solve the following problem :
It is observed that it rains on 10 days out of 30 days. Find the probability that it rains on exactly 3 days of a week.
A random variable X has the following probability distribution
| X | 2 | 3 | 4 |
| P(x) | 0.3 | 0.4 | 0.3 |
Then the variance of this distribution is
Let X be a discrete random variable whose probability distribution is defined as follows:
P(X = x) = `{{:("k"(x + 1), "for" x = 1"," 2"," 3"," 4),(2"k"x, "for" x = 5"," 6"," 7),(0, "Otherwise"):}`
where k is a constant. Calculate E(X)
For the following probability distribution:
| X | 1 | 2 | 3 | 4 |
| P(X) | `1/10` | `3/10` | `3/10` | `2/5` |
E(X2) is equal to ______.
A box contains 30 fruits, out of which 10 are rotten. Two fruits are selected at random one by one without replacement from the box. Find the probability distribution of the number of unspoiled fruits. Also find the mean of the probability distribution.
