Advertisements
Advertisements
प्रश्न
How will you find a point equidistant from three given points A, B, C which are not in the same straight line?
उत्तर
(i) The locus of points equidistant from three given points A, B & C is the straight line PQ, which bisects AB at right angles.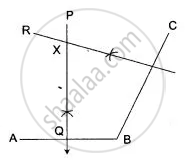
(ii) Similarly, the locus of points equidistant from B and C is the straight line RS which bisects BC at right angles.
Hence, the point common to PQ and RS must satisfy both conditions; that is to say, X the point of intersection of PQ and RS will be equidistant from A, B and C.
APPEARS IN
संबंधित प्रश्न
O is a fixed point. Point P moves along a fixed line AB. Q is a point on OP produced such that OP = PQ. Prove that the locus of point Q is a line parallel to AB.
Plot the points A(2, 9), B(–1, 3) and C(6, 3) on graph paper. On the same graph paper draw the locus of point A so that the area of ΔABC remains the same as A moves.
Without using set squares or protractor, construct a quadrilateral ABCD in which ∠ BAD = 45° , AD = AB = 6 cm, BC= 3.6 cm and CD=5 cm. Locate the point P on BD which is equidistant from BC and CD.
In given figure 1 ABCD is an arrowhead. AB = AD and BC = CD. Prove th at AC produced bisects BD at right angles at the point M

In Δ PQR, bisectors of ∠ PQR and ∠ PRQ meet at I. Prove that I is equidistant from the three sides of the triangle , and PI bisects ∠ QPR .
Draw and describe the lorus in the following cases:
The Iocus of the mid-points of all parallel chords of a circle.
Draw and describe the lorus in the following cases:
The lorus of a point in rhombus ABCD which is equidistant from AB and AD .
Describe completely the locus of point in the following cases:
Midpoint of radii of a circle.
Use ruler and compasses only for this question. Draw a circle of radius 4 cm and mark two chords AB and AC of the circle of length f 6 cm and 5 cm respectively.
(i) Construct the locus of points, inside the circle, that are equidistant from A and C. Prove your construction.
(ii) Construct the locus of points, inside the circle, that are equidistant from AB and AC.
Use ruler and compasses only for the following questions:
Construct triangle BCP, when CB = 5 cm, BP = 4 cm, ∠PBC = 45°.
Complete the rectangle ABCD such that :
(i) P is equidistant from AB and BC and
(ii) P is equidistant from C and D. Measure and write down the length of AB.
