Advertisements
Advertisements
प्रश्न
If an angle of a parallelogram is two-third of its adjacent angle, find the angles of the parallelogram .
उत्तर
Let the measure of the angle be x
∴ The measure of the angle adjacent is `(2x)/3`
We know that the adjacent angle of a parallelogram is supplementary
Hence x + `(2x) / 3` = 180°
2x + 3x = 540°
⇒ 5x = 540°
⇒ x = 108°
Adjacent angles are supplementary
⇒ x +108° = 180°
⇒ x =180° -108° = 72°
⇒ x = 72°
Hence, four angles are : 180°, 72°,108°, 72°
APPEARS IN
संबंधित प्रश्न
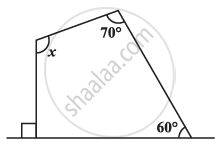
Find the angle measure x in the given Figure
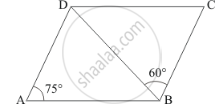
In Fig. below, ABCD is a parallelogram in which ∠DAB = 75° and ∠DBC = 60°. Compute
∠CDB and ∠ADB.
PQRS is a square such that PR and SQ intersect at O. State the measure of ∠POQ.
If PQRS is a square, then write the measure of ∠SRP.
The diagonals of a rectangle ABCD meet at O, If ∠BOC = 44°, find ∠OAD.
If the degree measures of the angles of quadrilateral are 4x, 7x, 9x and 10x, what is the sum of the measures of the smallest angle and largest angle?
Can all the angles of a quadrilateral be acute angles? Give reason for your answer.
If three angles of a quadrilateral are each equal to 75°, the fourth angle is ______.
The angles of a quadrilateral are in the ratio 1 : 2 : 3 : 4. The smallest angle is ______.
Sum of all the angles of a quadrilateral is 180°.
