Advertisements
Advertisements
प्रश्न
If the area of an equilateral triangle is `36sqrt3 cm^2` find its perimeter.
उत्तर
Area of equilateral triangle =`36sqrt3 cm^2`
Area of equilateral triangle=`(sqrt3/4xxa^2)` where a is the length of the side
⇒`36sqrt3=sqrt3/4xxa^2`
⇒`144=a^2`
⇒ `a=12 cm`
Perimeter of a triangle =`3a`
=`3xx12`
=`36cm`
APPEARS IN
संबंधित प्रश्न
Find the area of the shaded region in the given figure, if PQ = 24 cm, PR = 7 cm and O is the centre of the circle. [Use Π = 22/7]
Find the area of the shaded region in the given figure, if radii of the two concentric circles with centre O are 7 cm and 14 cm respectively and ∠AOC = 40° [Use Π = 22/7]

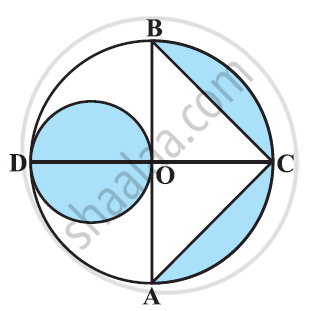
In the given figure, ABCD is a square of side 14 cm. With centres A, B, C and D, four circles are drawn such that each circle touches externally two of the remaining three circles. Find the area of the shaded region. [Use Π = 22/7]

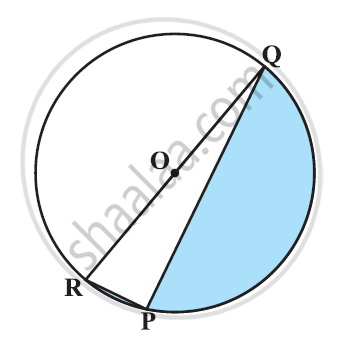
In the given figure, AB and CD are two diameters of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region. [Use Π = 22/7]
The radii of two circles are 19cm and 9 cm respectively. Find the radius and area of the circle which has circumferences is equal to sum of circumference of two circles.
The length of the two sides of a right triangle containing the right angle differ by 2 cm. If the area of the triangle is 24xm^2, find the perimeter of the triangle.
Each side of an equilateral triangle is 10 cm. Find (i) the area of the triangle and (ii) the height of the triangle.
The height of an equilateral triangle is 6 cm. Find its area.
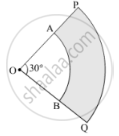
In Fig. 7, PQ and AB are respectively the arcs of two concentric circles of radii 7 cm and
3.5 cm and centre O. If ∠POQ = 30°, then find the area of the shaded region. [User`22/7`]
The area of the square that can be inscribed in a circle of radius 8 cm is ______.
