Advertisements
Advertisements
प्रश्न
The height of an equilateral triangle is 6 cm. Find its area.
उत्तर
Let the side of the equilateral triangle be x cm.
As, the area of an equilateral triangle = `sqrt3/4 "(side)"^2=(x^2sqrt3)/4`
Also, the area of the triangle =`1/2xxBasexxHeight=1/2xx x xx6=3x`
So, `(x^2sqrt3)/4=3x`
⇒` (xsqrt3)/4=3`
⇒`x=12/sqrt3`
⇒`x=12/sqrt3xxsqrt3/sqrt3`
⇒`x=(12sqrt3)/3`
⇒` x=4sqrt3 cm`
Now, area of the equilateral triangle =3x
=`3xx4sqrt3`
=`12sqrt3`
=`12xx1.73`
=`20.76 cm^2`
APPEARS IN
संबंधित प्रश्न
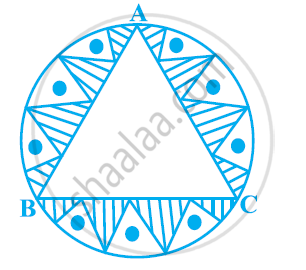
In a circular table cover of radius 32 cm, a design is formed leaving an equilateral triangle ABC in the middle as shown in the given figure. Find the area of the design (Shaded region). [Use Π = 22/7]
In the given figure, ABCD is a square of side 14 cm. With centres A, B, C and D, four circles are drawn such that each circle touches externally two of the remaining three circles. Find the area of the shaded region. [Use Π = 22/7]

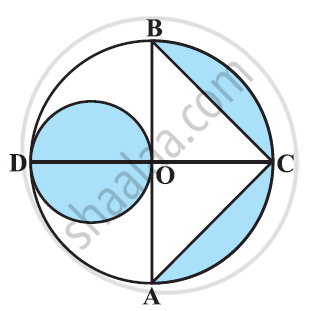
In the given figure, AB and CD are two diameters of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region. [Use Π = 22/7]
In the given figure, OACB is a quadrant of circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the
(i) Quadrant OACB
(ii) Shaded region
[Use Π = 22/7]

Calculate the area of the designed region in the given figure common between the two quadrants of circles of radius 8 cm each. [Use Π = 22/7]

If a square is inscribed in a circle, find the ratio of areas of the circle and the square.
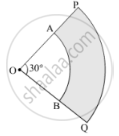
In Fig. 7, PQ and AB are respectively the arcs of two concentric circles of radii 7 cm and
3.5 cm and centre O. If ∠POQ = 30°, then find the area of the shaded region. [User`22/7`]
Find the area of triangle formed by joining the mid-points of the sides of the triangle whose vertices are A(2, 1), B(4, 3) and C(2, 5).
A plane left 30 minutes later than the scheduled time and in order to reach its destination 1500 km away on time, it has to increase its speed by 250 km/hr from its usual speed. Find the usual speed of the plane.
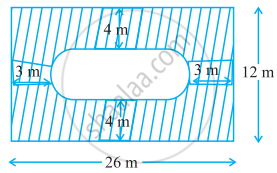
Find the area of the shaded region in figure.