Advertisements
Advertisements
प्रश्न
If the bar magnet is turned around by 180°, where will the new null points be located?
उत्तर
The magnetic field on the axis of the magnet at a distance d1 = 14 cm, can be written as:
`"B"_1 = (μ_0 2"M")/(4pi ("d"_1)^3) = "H"` .......(1)
Where,
M = Magnetic moment
μ0 = Permeability of free space
H = Horizontal component of the magnetic field at d1
If the bar magnet is turned through 180°, then the neutral point will lie on the equatorial line.
Hence, the magnetic field at a distance d2, on the equatorial line of the magnet can be written as:
`"B"_2 = (μ_0 "M")/(4pi ("d"_2)^3) = "H"` .............(2)
Equating equations (1) and (2), we get:
`2/("d"_1)^3 = 1/("d"_2)^3`
`("d"_2/"d"_1)^3 = 1/2`
∴ `"d"_2 = "d"_1 xx (1/2)^(1/3)`
= 14 × 0.794
= 11.1 cm
The new null points will be located 11.1 cm on the normal bisector.
APPEARS IN
संबंधित प्रश्न
Magnetic lines of force are closed continuous curves.
How are the magnetic field lines different from the electrostatic field lines?
Predict the polarity of the capacitor in the situation described below :

An iron needle is attracted to the ends of a bar magnet but not to the middle region of the magnet. Is the material making up the ends of a bare magnet different from that of the middle region?
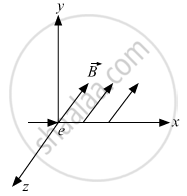
An electron moves along +x direction. It enters into a region of uniform magnetic field. `vecB` directed along –z direction as shown in fig. Draw the shape of the trajectory followed by the electron after entering the field.
Answer the following question in detail.
A circular magnet is made with its north pole at the centre, separated from the surrounding circular south pole by an air gap. Draw the magnetic field lines in the gap.
A closely wound solenoid of 800 turns and area of cross-section 2.5 × 10–4 m2 carries a current of 3.0 A. Explain the sense in which the solenoid acts like a bar magnet. What is its associated magnetic moment?
Which of the following statements about bar magnet is correct?
When iron filings are sprinkled on a sheet of glass placed over a short bar magnet then, the iron filings form a pattern suggesting that the magnet has ______.
According to the dipole analogy 1/ε0 corresponds to ______.
Magnetic moment for solenoid and corresponding bar magnet is ______.
Four point masses, each of value m, are placed at the comers of a square ABCD of side L, the moment of inertia of this system about an axis through A and parallel to BD is ______.
A particle having charge 100 times that of an electron is revolving in a circular path by radius 0.8 with one rotation per second. The magnetic field produced at the centre is
A bar magnet of magnetic moment m and moment of inertia I (about centre, perpendicular to length) is cut into two equal pieces, perpendicular to length. Let T be the period of oscillations of the original magnet about an axis through the midpoint, perpendicular to length, in a magnetic field B. What would be the similar period T′ for each piece?
Use (i) the Ampere’s law for H and (ii) continuity of lines of B, to conclude that inside a bar magnet, (a) lines of H run from the N pole to S pole, while (b) lines of B must run from the S pole to N pole.
Verify the Ampere’s law for magnetic field of a point dipole of dipole moment m = m`hatk`. Take C as the closed curve running clockwise along (i) the z-axis from z = a > 0 to z = R; (ii) along the quarter circle of radius R and centre at the origin, in the first quadrant of x-z plane; (iii) along the x-axis from x = R to x = a, and (iv) along the quarter circle of radius a and centre at the origin in the first quadrant of x-z plane.
