Advertisements
Advertisements
प्रश्न
If the diagonals of a rhombus get doubled, then the area of the rhombus becomes ______ its original area.
उत्तर
If the diagonals of a rhombus get doubled, then the area of the rhombus becomes 4 times its original area.
Explanation:
We know that,
Area of a rhombus = `1/2 xx d_1 xx d_2`
Where, d1 and d2 are diagonals of the rhombus.
If diagonals get doubled, then the area = `1/2 2d_1 xx 2d_2 = 4(1/2 xx d_1 xx d_2)`
Hence, the new area becomes 4 times its original area.
APPEARS IN
संबंधित प्रश्न
The length of the diagonals of a rhombus is in ratio 4 : 3. If its area is 384 cm2, find its side.
The area of a rhombus is equal to the area of a triangle. If the base ∆ is 24 cm, its corresponding altitude is 16 cm and one of the diagonals of the rhombus is 19.2 cm. Find its other diagonal.
Find the area of a rhombus whose diagonals are of lengths 10 cm and 8.2 cm.
Find the area of rhombus PQRS shown in the following figure.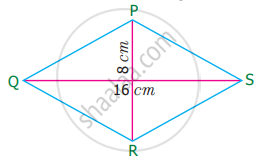
Find the area of rhombus PQRS shown in the following figure.
Find the area of a rhombus whose base is 14 cm and height is 9 cm.
The area of the rhombus with side 4 cm and height 3 cm is
The height of the rhombus whose area 96 sq.m and side 24 m is
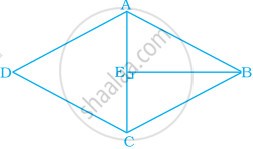
What is the area of the rhombus ABCD below if AC = 6 cm and BE = 4 cm?
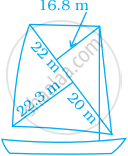
Most of the sailboats have two sails, the jib and the mainsail. Assume that the sails are triangles. Find the total area sail of the sailboats to the nearest tenth.