Advertisements
Advertisements
प्रश्न
In an agricultural experiment, a solution containing 1 mole of a radioactive material (t1/2= 14.3 days) was injected into the roots of a plant. The plant was allowed 70 hours to settle down and then activity was measured in its fruit. If the activity measured was 1 µCi, what per cent of activity is transmitted from the root to the fruit in steady state?
उत्तर
Given:
Initial no of atoms, N0 = 1 mole = 6 × 1023 atoms
Half-life of the radioactive material, T1/2 = 14.3 days
Time taken by the plant to settle down, t = 70 h
Disintegration constant, `lambda = 0.693/t_"1/2"` = `0.693/(14.3 xx 24) "h"^-1`
`N = N_0e^(-lambdat)`
= `6 xx 10^23 xx e^((-0.693 xx 70)/(14.3 xx 24))`
= `6 xx 10^23 xx 0.868`
= `5.209 xx 10^23`
Activity , `R = "dN"/"dt" = 5.209 xx 10^23 xx 0.693/(14.3 xx 24)`
= `(0.0105 xx 10^23)/3600 "dis/hr"`
= `2.9 xx 10^-6 xx 10^23 "dis/sec"`
= `2.9 xx 10^17 "dis/sec"`
Fraction of activity transmitted = `((1 µCi)/(2.9 xx 10^17)) xx 100%`
= `[((1 xx 3.7 xx 10^4)/(2.9 xx 10^17)) xx 100%]`
= `1.275 xx 10^-11 %`
APPEARS IN
संबंधित प्रश्न
The half life of a certain radioactive material against \u0003α-decay is 100 days. After how much time, will the undecayed fraction of the material be 6.25%?
Write nuclear reaction equation for β−-decay of `""_83^210"Bi"`.
Plot a graph showing variation of activity of a given radioactive sample with time.
The sequence of stepwise decay of a radioactive nucleus is
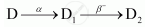
If the atomic number and mass number of D2 are 71 and 176 respectively, what are their corresponding values of D?
State the law of radioactive decay. hence derive the relation N = Noe-λt . Represent it graphically.
The half-life of 199Au is 2.7 days. (a) Find the activity of a sample containing 1.00 µg of 198Au. (b) What will be the activity after 7 days? Take the atomic weight of 198Au to be 198 g mol−1.
The half-life of a radioisotope is 10 h. Find the total number of disintegration in the tenth hour measured from a time when the activity was 1 Ci.
4 × 1023 tritium atoms are contained in a vessel. The half-life of decay tritium nuclei is 12.3 y. Find (a) the activity of the sample, (b) the number of decay in the next 10 hours (c) the number of decays in the next 6.15 y.
238U decays to 206Pb with a half-life of 4.47 × 109 y. This happens in a number of steps. Can you justify a single half for this chain of processes? A sample of rock is found to contain 2.00 mg of 238U and 0.600 mg of 206Pb. Assuming that all the lead has come from uranium, find the life of the rock.
A charged capacitor of capacitance C is discharged through a resistance R. A radioactive sample decays with an average-life τ. Find the value of R for which the ratio of the electrostatic field energy stored in the capacitor to the activity of the radioactive sample remains constant in time.
Radioactive isotopes are produced in a nuclear physics experiment at a constant rate dN/dt = R. An inductor of inductance 100 mH, a resistor of resistance 100 Ω and a battery are connected to form a series circuit. The circuit is switched on at the instant the production of radioactive isotope starts. It is found that i/N remains constant in time where i is the current in the circuit at time t and N is the number of active nuclei at time t. Find the half-life of the isotope.
`""_83^212"Bi"` can disintegrate either by emitting an α-particle of by emitting a β−-particle. (a) Write the two equations showing the products of the decays. (b) The probabilities of disintegration α-and β-decays are in the ratio 7/13. The overall half-life of 212Bi is one hour. If 1 g of pure 212Bi is taken at 12.00 noon, what will be the composition of this sample at 1 P.m. the same day?
Plot a graph showing the variation of undecayed nuclei N versus time t. From the graph, find out how one can determine the half-life and average life of the radioactive nuclei.
A radioactive substance decays to 1/16th of its initial mass in 40 days. The half-life of the substance, in days, is:
The half-life of a certain radioactive element is 3.465 days. Find its disintegration constant.
Half-life of a certain radioactive material is 8 hours.
Find the disintegration constant of this material.
Half life of a certain radioactive material is 8 hours.
If one starts with 600 g of this substance, how much of it will disintegrate in one day?
A nucleus with Z = 92 emits the following in a sequence:
α, β‾, β‾, α, α, α, α, α, β‾, β‾, α, β+, β+, α
Then Z of the resulting nucleus is ______.
