Advertisements
Advertisements
प्रश्न
In the below fig, transversal 𝑙 intersects two lines m and n, `∠`4 = 110° and `∠`7 = 65°. Is m || n?

उत्तर १
Given:
`∠`4 = 110°, `∠`7 = 65°
To find: Is m || n
Here, `∠`7 =`∠`5= 65° [Vertically opposite angle]
Now,
`∠`4 + `∠`5 = 110 + 65° = 175°
∴m is not parallel to n as the pair of co-interior angles is not supplementary.
उत्तर २
The figure is given as follows:
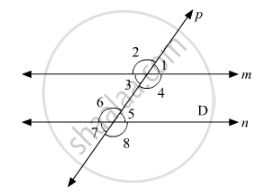
It is given that l is a transversal to lines m and n. Also,
∠4 = 110° and ∠7 = 65°.
We need check whether m||n or not.
We have.∠7 = 65°.
Also,∠7 5.and ∠5are vertically opposite angles, thus, these two must be equal. That is,
∠5 = °65.
Also, ∠4 =110°
Adding this equation to (i), we get:
∠4 + ∠5 =110° +65°
∠4 +∠5 =175°
But these are the consecutive interior angles which are not supplementary.
Theorem states: If a transversal intersects two lines in such a way that a pair of consecutive interior angles is supplementary, then the two lines are parallel.
Thus, m is not parallel to n.
APPEARS IN
संबंधित प्रश्न
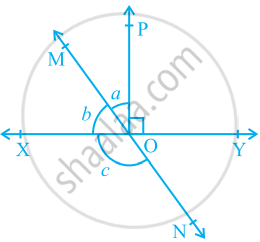
In the given figure, lines XY and MN intersect at O. If ∠POY = 90° and a : b = 2 : 3, find c.
Write the supplement of the following angles .
138°
Two supplementary angles differ by 48°. Find the angles.
If an angle differs from its complement by 10°, find the angle .
If the supplement of an angle is two-third of itself. Determine the angle and its supplement.
In the below Fig, OA and OB are opposite rays.
If y = 35°, what is the value of x?
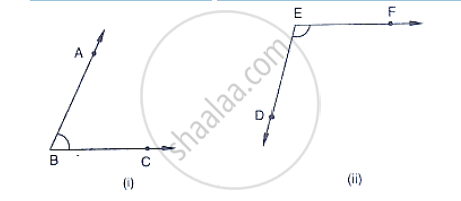
In the below fig, write all pairs of adjacent angles and all the linear pairs .

If below fig, ∠AOF and ∠FOG form a linear pair.
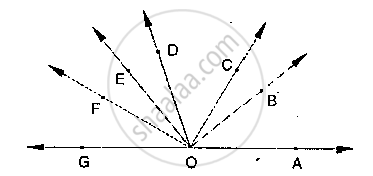
∠EOB = ∠FOC = 90° and ∠DOC = ∠FOG = ∠AOB = 30°
(i) Find the measures of ∠FOE, ∠COB and ∠DOE.
(ii) Name all the right angles.
(iii) Name three pairs of adjacent complementary angles.
(iv) Name three pairs of adjacent supplementary angles.
(v) Name three pairs of adjacent angles.
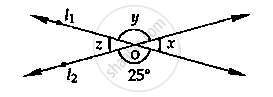
In the given fig, find the values of x, y and z.
In the below fig, arms BA and BC of ∠ABC are respectively parallel to arms ED and EF of
∠DEF. Prove that ∠ABC + ∠DEF = 180°.