Advertisements
Advertisements
प्रश्न
In ∆DEF, DN, EO, FM are medians and point P is the centroid. Find the following
If OE = 36 then EP = ?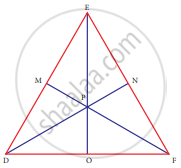
उत्तर
Given DN, EO, FM are medians.
∴ FN = EN
DO = FO
EM = DM
If OE = 36
then `"EP"/"PO" = 2/1`
`"EP"/2` = PO
OE = OP + PE
36 = `"PE"/2 + "PE"`
36 = `"PE"/2 + (2"PE")/2`
36 = `(3"PE")/2`
PE = `(36 xx 2)/3`
PE = 24
APPEARS IN
संबंधित प्रश्न
The length of hypotenuse of a right angled triangle is 15. Find the length of median of its hypotenuse.
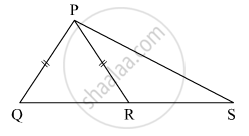
In the given figure, if seg PR ≅ seg PQ, show that seg PS > seg PQ.
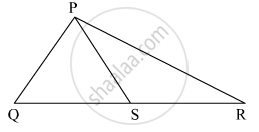
In the given figure, point S is any point on side QR of ΔPQR Prove that: PQ + QR + RP > 2PS
Draw an isosceles triangle. Draw all of its medians and altitudes. Write your observation about their points of concurrence.
The medians of a triangle cross each other at _______
In any triangle the centroid and the incentre are located inside the triangle
The centroid, orthocentre, and incentre of a triangle are collinear
In the given figure, A is the midpoint of YZ and G is the centroid of the triangle XYZ. If the length of GA is 3 cm, find XA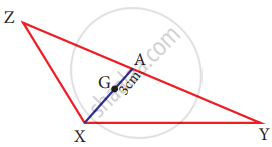
In ∆DEF, DN, EO, FM are medians and point P is the centroid. Find the following
If DO = 8, then FD = ?
In ∆ABC, AD is the bisector of ∠A meeting BC at D, CF ⊥ AB and E is the mid-point of AC. Then median of the triangle is ______.
