Advertisements
Advertisements
प्रश्न
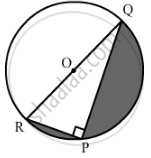
In the given figure, PQ = 24, PR = 7 cm and O is the centre of the circle. Find the area of the shaded region.
उत्तर
In the right ΔRPQ, we have:
`"RQ" = sqrt("RP"^2 + "PQ"^2)`
`=sqrt(7^2+24^2)`
`= sqrt(49+576)`
= 25 cm
OR = OQ = 12.5 cm
Now,
Area of the circle = πr2
= 3.14 × 12.5 × 12.5
= 490.625 sq. cm
Area of the semicircle `= 490.625/2 = 245.31 "sq.cm" `
Area of the traingle `= 1/2xx"b"xx"h" = 1/2xx7xx24=84 "sq.cm"`
Thus, we have
Area of the shaded part = Area of the semicircle - Area of the triangle]
= 245.13 - 84
= 161.31 cm2
APPEARS IN
संबंधित प्रश्न
Find the area enclosed between two concentric circles of radii 3.5 cm and 7 cm. A third concentric circle is drawn outside the 7 cm circle , such that the area enclosed between it and the 7 cm circle is same as that between the two inner circles . Find the radius of the third circle correct to one decimal place.
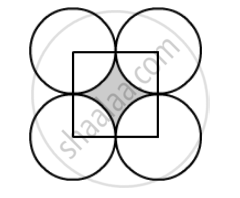
The diameter of a coin is 1 cm (in the following figure). If four such coins be placed on a table so that the rim of each touches that of the other two, find the area of the shaded region (Take π = 3.1416).
If the numerical value of the area of a circle is equal to the numerical value of its circumference , find its radius.
If the sum of the areas of two circles with radii r1 and r2 is equal to the area of a circle of radius r, then \[r_1^2 + r_2^2\]
The area of the sector of a circle of radius 10.5 cm is 69.3 cm2. Find the central angle of the sector.
The length of the minute hand of a clock is 21 cm. The area swept by the minute hand in 10 minutes is
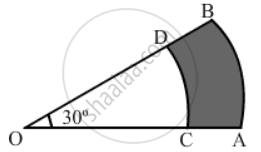
In the given figure, the sectors of two concentric circles of radii 7 cm and 3.5 cm are shown. Find the area of the shaded region.
A square tank has an area of 1600 cm2. There are four semicircular plots around it. Find the cost of turfing the plots at Rs 12.50 per m2
How much distance, in metres, a wheel of 25 cm radius will cover if it rotates 350 times?
Area of a quadrant of a circle of radius 7 cm is ______.
