Advertisements
Advertisements
प्रश्न
In the given figure, the side of square is 28 cm and radius of each circle is half of the length of the side of the square where O and O' are centres of the circles. Find the area of shaded region.
उत्तर
We have,
Side of square = 28 cm and radius of each circle = `28/2` cm
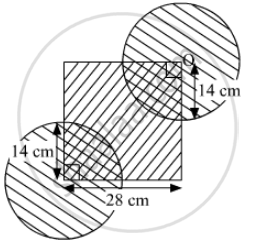
Area of the shaded region
= Area of the square + Area of the two circles − Area of the two quadrants
`= (28)^2 + 2 xx pi xx (28/2)^2 - 2 xx 1/4 xx pi xx (28/2)^2`
`= (28)^2 + 3/2 xx pi xx (28/2)^2`
`= (28)^2 (1 + 3/2 xx 22/7 xx 1/2 xx 1/2)`
`= (28)^2 xx 61/28`
`= 28 xx 61`
`= 1708 cm^2`
Therefore, the area of the shaded region is 1708 cm2
APPEARS IN
संबंधित प्रश्न
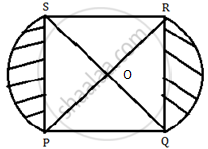
In the figure given below, PQRS is square lawn with side PQ = 42 metres. Two circular flower beds are there on the sides PS and QR with centre at O, the intersections of its
diagonals. Find the total area of the two flower beds (shaded parts).
The circumference of a circle exceeds its diameter by 45 cm. Find the circumference of the circle.
If the perimeter of a square is equal to the circumference of a circle then the ratio of their areas is
In a circle of radius 14 cm, an arc subtends an angle of 120° at the centre. If `sqrt(3) = 1.73` then the area of the segment of the circle is
Find the area and perimeter of the following semi-circle :
Radius= 1.4 cm
A solid sphere metal is cut through its centre into 2 equal parts. If the diameter of the sphere is 3`1/3` cm , find the total surface of each part, correct to two decimal places.
A metal wire, when bent in the form of an equilateral triangle of largest area, encloses an area of 484 `sqrt3` cm2. If the same wire is bent into the form of a circle of largest area, find the area of this circle.
The radii of two circles are 48 cm and 13 cm. Find the area of the circle which has its circumference equal to the difference of the circumferences of the given two circles.
Diameters of different circles are given below. Find their circumference (Take π = `22/7`)
d = 28 mm
