Advertisements
Advertisements
प्रश्न
In the diagram given alongside, AC is the diameter of the circle, with centre O. CD and BE are parallel. ∠ AOB = 80° and ∠ ACE = 10°. Calculate: (i) ∠ BEC (ii) ∠ BCD (iii) ∠ CED.

उत्तर
From the figure, we have
∠ AOB = 80°
∠ ACE = 10°
(i) ∠ BOC = 180° - ∠ AOB
⇒ ∠ BOC = 180° - 80°
⇒ ∠ BOC = 100°
∠ BEC = `1/2`∠ BOC ...( ∵ ∠ Subtended at the centre and ∠ subtend by E by arc BC)
⇒ ∠ BEC = `1/2` x 100°
⇒ ∠ BEC = 50°
(ii) ∠ ACB = `1/2` ∠ AOB ...( ∵ ∠s Subtended by arc AB at the centre at C)
⇒ ∠ ACB = `1/2 xx 80°`
⇒ ∠ ACB = 40°
∠ ECD = ∠ BEC ...( ∵ Alternate ∠s as CD || BE)
∠ ECD = 50°
⇒ ∠ BCD = ∠ ACB + ∠ ECA + ∠ ECD
⇒ ∠ BCD = 40° + 10° + 50°
⇒ ∠ BCD = 100°
(iii) BCDE is a cyclic quadrilateral, ....(∵ Its opposite ∠s are supplementary)
⇒ ∠ BED = 180° - ∠ BCD
⇒ ∠ BED = 180° - 100° ....( From ii)
⇒ ∠ BED = 80°
⇒ ∠ BEC + ∠ CED = 80°
⇒ ∠ CED = 80° - ∠ BED
⇒ ∠ CED = 80° - 50°
⇒ ∠ CED = 30° ....( From i)
APPEARS IN
संबंधित प्रश्न
In the figure given below AB and CD are two parallel chords and O is the centre. If the radius of the circle is 15 cm, find the distance MN between the two chords of length 24 cm and 18 cm respectively.

AB and CD are two equal chords of a circle with centre O which intersect each other at right
angle at point P. If OM⊥ AB and ON ⊥ CD; show that OMPN is a square.
In the following figure, O is the centre of the circle. Find the values of a, b and c.

In the following figure, O is the centre of the circle. Find the values of a, b, c and d

Calculate:
- ∠CDB,
- ∠ABC,
- ∠ACB.
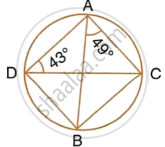
Given: ∠CAB = 75° and ∠CBA = 50°. Find the value of ∠DAB + ∠ABD.

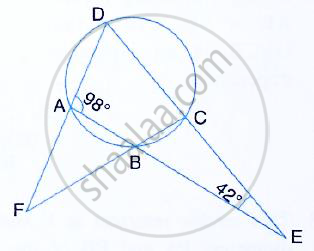
The sides AB and DC of a cyclic quadrilateral ABCD are produced to meet at E; the sides DA and CB are produced to meet at F. If ∠BEC = 42° and ∠BAD = 98°; Calculate :
(i) ∠AFB (ii) ∠ADC
The line joining the midpoints of two chords of a circle passes through its center.
Prove that the chords are parallel.
AB and CD are two chords of a circle such that AB = 6 cm, CD = 12 cm and AB || CD. If the distance between AB and CD is 3 cm, find the radius of the circle.
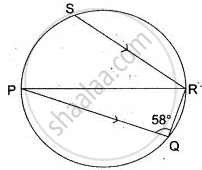
In the adjoining figure, PQ is the diameter, chord SR is parallel to PQ. Give ∠ PQR = 58°.
Calculate:
(i) ∠ RPQ,
(ii) ∠ STP ( T is a point on the minor arc)