Advertisements
Advertisements
प्रश्न
In triangle PQR, PQ = 24 cm, QR = –7 cm and ∠PQR = 90°. Find the radius of the inscribed circle.

उत्तर १
Since ΔPQR is a right-angled angle,
PR = `sqrt(7^2 + 24^2) = sqrt(49 + 576) = sqrt625 = 25 cm`
Let the given inscribed circle touches the sides of the given triangle at points A, B and C respectively.
Then, clearly, OAQB is a square.
=> AQ = BQ = x cm
PA = PQ – AQ = (24 – x) cm
RB = QR – BQ = (7 – x) cm
Since tangents from an exterior point to a circle are equal,
PC = PA = (24 – x) cm
And, RC = RB = (7 – x) cm
PR = PC + CR
=> 25 = (24 – x) + (7 – x)
=> 25 = 31 – 2x
=> 2x = 6
=> x = 3 cm
Hence, the radius of the inscribed circle is 3 cm.
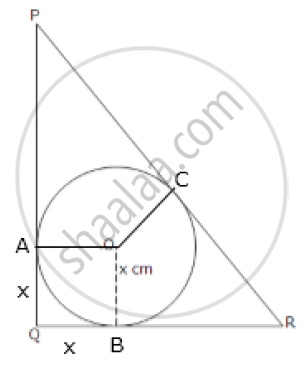
उत्तर २
OM ⊥ QR
ON ⊥ PQ ....( Tangents and radius perpendicular to each other.)
OM = ON = r
QM = QN ....( Tangents from an external point)

⇒ QMON is a square.
⇒ QM = OM = ON = QN = x cm
So, mR = (7 - x) cm
PN = (24 - x) cm
PT = PN = 24 - x
and, mR = RT = 7 - x ....(Tangents from an external point)
⇒ PR = PT + RT
PR = 24 - x + 7 - x
PR = 31 - 2x
Now, In ΔPQR,
PR2 = PQ2 + QR2
PR2 = 242 + 72
PR2 = 576 + 49 = 625
PR = 25 cm
⇒ 31 - 2x = 25
⇒ 2x = 31 - 25
⇒ 2x = 6
⇒ x = 3.
APPEARS IN
संबंधित प्रश्न
In the given figure O is the centre of the circle and AB is a tangent at B. If AB = 15 cm and AC = 7.5 cm. Calculate the radius of a circle.

The given figure shows a circle with centre O and BCD is tangent to it at C. Show that : ∠ACD + ∠BAC = 90°.

If Δ ABC is isosceles with AB = AC and C (O, r) is the incircle of the ΔABC touching BC at L,prove that L bisects BC.
A point A is 17cm from the centre of the circle. The length of the tangent drawn from A to the circle is 15cm. find the radius of the circle.
Draw a circle of radius 2.7 cm and draw a chord PQ of length 4.5 cm. Draw tangents at points P and Q without using centre.
ΔABC circumscribes a circle of radius r such that ∠B = 90°. If AB = 3 cm and BC = 4 cm, then find the value of r.
The distance between two tangents parallel to each other of a circle is 13 cm. Find the radius of the circle.
Assertion (A): A tangent to a circle is perpendicular to the radius through the point of contact.
Reason (R): The lengths of tangents drawn from an external point to a circle are equal.
In the adjoining figure, PT is a tangent at T to the circle with centre O. If ∠TPO = 30°, find the value of x.

If O is centre of a circle and Chord PQ makes an angle 50° with the tangent PR at the point of contact P, then the angle subtended by the chord at the centre is ______.