Advertisements
Advertisements
प्रश्न
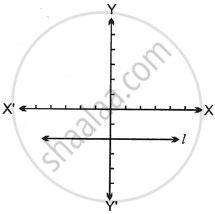
In the figure, line l is parallel to X-axis. Which of the following statement is true?
विकल्प
The slope is zero.
The slope cannot be determined.
The slope is positive.
The slope is negative.
उत्तर
The slope is zero.
Explanation:
The slope of a line parallel to the X-axis is zero.
APPEARS IN
संबंधित प्रश्न
Find the slope of the line with inclination 30° .
Find the slope of the line passing through the points A(-2, 1) and B(0, 3).
The slope of a line joining P(6, k) and Q(1 – 3k, 3) is `1/2`. Find:
- k.
- mid-point of PQ, using the value of ‘k’ found in (i).
(−2, 4), (4, 8), (10, 7) and (11, –5) are the vertices of a quadrilateral. Show that the quadrilateral, obtained on joining the mid-points of its sides, is a parallelogram.
Find x, if the slope of the line joining (x, 2) and (8, −11) is `−3/4`.
The side AB of a square ABCD is parallel to the x-axis. Find the slopes of all its sides. Also, find:
- the slope of the diagonal AC.
- the slope of the diagonal BD.
Determine x so that the slope of the line through (1, 4) and (x, 2) is 2.
Determine whether the following point is collinear.
P(2, –5), Q(1, –3), R(–2, 3)
Fill in the blank using correct alternative.
Seg AB is parallel to Y-axis and coordinates of point A are (1,3) then co–ordinates of point B can be ........ .
Fill in the blank using correct alternative.
A line makes an angle of 30° with the positive direction of X– axis. So the slope of the line is ______.
Show that the line joining the points A(4, 8) and B(5, 5) is parallel to the line joining the points C(2, 4) and D(1, 7).
Find the slope of a line, correct of two decimals, whose inclination is 50°
Find the slope of a line passing through the following pair of points
(5pq,p2q) and (5qr,qr2)
Find slope of a line passing through the points A(3, 1) and B(5, 3).
Find the slope of a line passing through the point A (-2,1), B (0,3).
Find the slope of the line passing through the points A(4,7) and B(2,3).
Show that points A(– 4, –7), B(–1, 2), C(8, 5) and D(5, – 4) are the vertices of a parallelogram ABCD
Determine whether the following points are collinear. A(–1, –1), B(0, 1), C(1, 3)
Given: Points A(–1, –1), B(0, 1) and C(1, 3)
Slope of line AB = `(square - square)/(square - square) = square/square` = 2
Slope of line BC = `(square - square)/(square - square) = square/square` = 2
Slope of line AB = Slope of line BC and B is the common point.
∴ Points A, B and C are collinear.
