Advertisements
Advertisements
प्रश्न
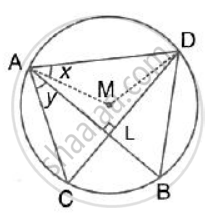
In the given figure, M is the centre of the circle. Chords AB and CD are perpendicular to each other. If ∠MAD = x and ∠BAC = y :
- express ∠AMD in terms of x.
- express ∠ABD in terms of y.
- prove that : x = y.

उत्तर
In the figure, M is the centre of the circle.
Chords AB and CD are perpendicular to each other at L.
∠MAD = x and ∠BAC = y
i. In ∆AMD,
MA = MD
∴ ∠MAD = ∠MDA = x
But in ∆AMD,
∠MAD + ∠MDA + ∠AMD = 180°
`=>` x + x + ∠AMD = 180°
`=>` 2x + ∠AMD = 180°
`=>` ∠AMD = 180° – 2x
ii. ∴ Arc AD∠AMD at the centre and ∠ABD at the remaining
(Angle in the same segment)
(Angle at the centre is double the angle at the circumference subtended by the same chord)
`=>` ∠AMD = 2∠ABD
`=> ∠ABD = 1/2 (180^circ - 2x)`
`=>` ∠ABD = 90° – x
AB ⊥ CD, ∠ALC = 90°
In ∆ALC,
∴ ∠LAC + ∠LCA = 90°
`=>` ∠BAC + ∠DAC = 90°
`=>` y + ∠DAC = 90°
∴ ∠DAC = 90° – y
We have, ∠DAC = ∠ABD [Angles in the same segment]
∴ ∠ABD = 90° – y
iii. We have, ∠ABD = 90° – y and ∠ABD = 90° – x [Proved]
∴ 90° – x = 90° – y
`=>` x = y
APPEARS IN
संबंधित प्रश्न
The figure shows two concentric circles and AD is a chord of larger circle.
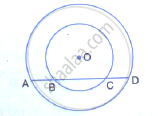
Prove that: AB = CD
In Δ ABC, the perpendicular from vertices A and B on their opposite sides meet (when produced) the circumcircle of the triangle at points D and E respectively. Prove that: arc CD = arc CE
In the given figure, O is the centre of the circle with radius 5 cm. OP and OQ are perpendicular to AB and CD respectively. AB = 8 cm and CD = 6 cm. determine the length of PQ.
Two chords of lengths 10cm and 24cm are drawn parallel o each other in a circle. If they are on the same side of the centre and the distance between them is 17cm, find the radius of the circle.
In the given figure, M is the centre of the circle. Chords AB and CD are perpendicular to each other.
If ∠MAD = x and ∠BAC = y , Prove that : x = y
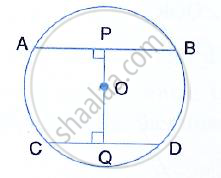
In the given figure, O is the center of the circle. AB and CD are two chords of the circle. OM is perpendicular to AB and ON is perpendicular to CD. AB = 24 cm, OM = 5 cm, ON = 12 cm, 
Find the :
(i) the radius of the circle
(ii) length of chord CD.
In a circle of radius 17 cm, two parallel chords of lengths 30 cm and 16 cm are drawn. Find the distance between the chords,
if both the chords are:
(i) on the opposite sides of the centre;
(ii) on the same side of the centre.
A chord of length 24 cm is at a distance of 5 cm from the center of the circle. Find the length of the chord of the same circle which is at a distance of 12 cm from the center.
In the following figure, AD is a straight line, OP ⊥ AD and O is the centre of both circles. If OA = 34cm, OB = 20 cm and OP = 16 cm;
find the length of AB.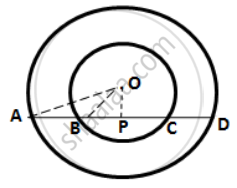
Find the length of a chord which is at a distance of 5 cm from the centre of a circle of radius 13 cm.
