Advertisements
Advertisements
प्रश्न
The given figure shows a circle with centre O and ∠ABP = 42°.

Calculate the measure of:
- ∠PQB
- ∠QPB + ∠PBQ
उत्तर
In the figure
∠ABP = 42°.
Join PO, QO
∵ Arc PA subtends ∠POA at the centre and
∵ ∠PBA at the remaining part.
∴ ∠POA = 2∠PBA = 2 × 42° = 84°
But ∠AOP + ∠BOP = 180° ...(Linear pair)
`\implies` ∠POA + ∠POB = 180°
`\implies` 84° + ∠POB = 180°
`\implies` POB = 180° – 84° = 96°
Similarly, arc BP subtrends ∠BOP on the centre and ∠PQB at the remaining part of the circle
∴ `∠PQB = 1/2`
`∠POB = 1/2 xx 96^circ = 48^circ`
But in ΔABQ,
∠QPB + ∠PBQ + ∠PQB = 180° ...(Angles of a triangle)
∠QPB + ∠PBQ + 48° = 180°
`\implies` ∠QPB + ∠PBQ = 180°
`\implies` ∠QPB + ∠PBQ = 180° – 48° = 132°
Hence i. PQB = 48° and ii. ∠QPB + ∠PBQ = 132°
APPEARS IN
संबंधित प्रश्न
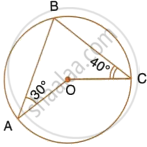
In the given figure, O is the centre of the circle. ∠OAB and ∠OCB are 30° and 40° respectively. Find ∠AOC . Show your steps of working.
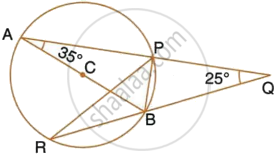
AB is a diameter of the circle APBR as shown in the figure. APQ and RBQ are straight lines. Find : ∠PRB
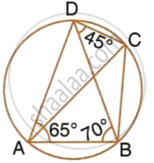
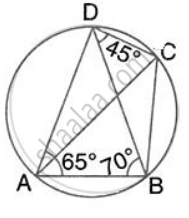
In the given figure, ∠BAD = 65°, ∠ABD = 70° and ∠BDC = 45°. Find:
- ∠BCD
- ∠ACB
Hence, show that AC is a diameter.
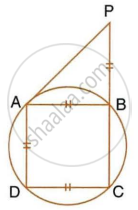
In the given figure, AB = AD = DC = PB and ∠DBC = x°. Determine, in terms of x :
- ∠ABD,
- ∠APB.
Hence or otherwise, prove that AP is parallel to DB.
In the given figure, ∠ACE = 43° and ∠CAF = 62°; find the values of a, b and c.

In the figure given alongside, AB and CD are straight lines through the centre O of a circle. If ∠AOC = 80° and ∠CDE = 40°, find the number of degrees in ∠ABC.

In the given figure, ∠BAD = 65°, ∠ABD = 70° and ∠BDC = 45°. Find: ∠ ACB.
Hence, show that AC is a diameter.
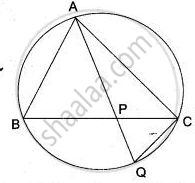
In the given Figure. P is any point on the chord BC of a circle such that AB = AP. Prove that CP = CQ.
In the given below the figure, AB is parallel to DC, ∠BCD = 80° and ∠BAC = 25°, Find
(i) ∠CAD, (ii) ∠CBD, (iii) ∠ADC.

In the given figure, ∠CAB = 25°, find ∠BDC, ∠DBA and ∠COB
