Advertisements
Advertisements
प्रश्न
Monica has a piece of Canvas whose area is 551 m2. She uses it to have a conical tent made, with a base radius of 7m. Assuming that all the stitching margins and wastage incurred while cutting, amounts to approximately 1 m2. Find the volume of the tent that can be made with it.
उत्तर
Given that,
Area of canvas ` 551m^2` and area of the canvas lost in wastage is `1m^2`
∴ area of canvas available for making the tent is `(551-1)m^2=550m^2.`
SA of tent =`550m^2` required base radius of conical tent= 7m
CSA of tent=`550m^2 `
`pirl=550m^2`
`⇒22/7xx7xxl=550`
`⇒l=550/22=25m `
Now, WKT
`l^2=r^2+h^2`
`⇒(25)^2-(7)^2=h^2 `
`⇒ h=sqrt(625-49)`
`=sqrt576=24m`
So, the volume of the conical tent =`1/3pir^2h`
`= 1/3xx3.14xx(7xx7)(24)m^3=1232m^3`
APPEARS IN
संबंधित प्रश्न
There are two cones. The curved surface area of one is twice that of the other. The slant height of the later is twice that of the former. Find the ratio of their radii.
A conical tent is 10 m high and the radius of its base is 24 m. Find the slant height of the tent. If the cost of 1 2 m canvas is Rs. 70, find the cost of the canvas required to make the tent.
Two cones have their heights in the ratio 1 : 3 and the radii of their bases in the ratio 3 : 1. Find the ratio of their volumes.
A solid cone of height 8 cm and base radius 6 cm is melted and recast into identical cones, each of height 2 cm and diameter 1 cm. Find the number of cones formed.
A cone of height 15 cm and diameter 7 cm is mounted on a hemisphere of same diameter. Determine the volume of the solid thus formed.
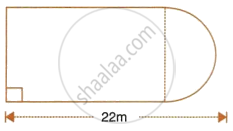
In the following diagram a rectangular platform with a semi-circular end on one side is 22 metres long from one end to the other end. If the length of the half circumference is 11 metres, find the cost of constructing the platform, 1.5 metres high at the rate of Rs. 4 per cubic metres.
The curved surface area of a cone is 2200 sq.cm and its slant height is 50 cm. Find the total surface area of cone. `(π = 22/7)`
A sphere and a cone have the same radii. If their volumes are also equal, prove that the height of the cone is twice its radius.
The volume of a conical tent is 1232 m3 and the area of the base floor is 154 m2. Calculate the: height of the tent.
A cloth having an area of 165 m2 is shaped into the form of a conical tent of radius 5 m. How many students can sit in the tent if a student, on an average, occupies `5/7` m2 on the ground?
