Advertisements
Advertisements
प्रश्न
Prove that the diagonals of a rectangle are perpendicular if and only if the rectangle is a square.
उत्तर
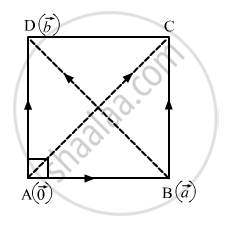
Let ABCD be a rectangle. Take A as the origin.
Suppose the position vectors of points B and D be \[\vec{a}\] and \[\vec{b}\] respectively.
Now,
\[\vec{AC} = \vec{AB} + \vec{BC} = \vec{AB} + \vec{AD} = \vec{a} + \vec{b}\]
Also,
\[\vec{BD} = \vec{a} - \vec{b}\]
Since ABCD is rectangle, so \[\vec{AB} \perp \vec{AD}\]
\[\therefore \vec{a} . \vec{b} = 0\]
Now, diagonals AC and BD are perpendicular iff \[\vec{AC} . \vec{BD} = 0\]
\[\text{ iff } \left( \vec{a} + \vec{b} \right) . \left( \vec{a} - \vec{b} \right) = 0\]
\[\text{ iff } \left| \vec{a} \right|^2 - \left| \vec{b} \right|^2 = 0\]
\[\text{ iff }\left| \vec{a} \right| = \left| \vec{b} \right|\]
\[\text{ iff } \left| \vec{AB} \right| = \left| \vec{AD} \right|\]
iff ABCD is a square
Thus, the diagonals of a rectangle are perpendicular if and only if the rectangle is a square.
APPEARS IN
संबंधित प्रश्न
Find the coordinate of the point P where the line through A(3, –4, –5) and B(2, –3, 1) crosses the plane passing through three points L(2, 2, 1), M(3, 0, 1) and N(4, –1, 0).
Also, find the ratio in which P divides the line segment AB.
In a triangle OAB,\[\angle\]AOB = 90º. If P and Q are points of trisection of AB, prove that \[{OP}^2 + {OQ}^2 = \frac{5}{9} {AB}^2\]
Let `A (bara)` and `B (barb)` are any two points in the space and `"R"(bar"r")` be a point on the line segment AB dividing it internally in the ratio m : n, then prove that `bar r = (mbarb + nbara)/(m + n) `
Find the position vector of point R which divides the line joining the points P and Q whose position vectors are `2hati - hatj + 3hatk` and `- 5hati + 2hatj - 5hatk` in the ratio 3:2 is internally.
Find the position vector of point R which divides the line joining the points P and Q whose position vectors are `2hat"i" - hat"j" + 3hat"k"` and `- 5hat"i" + 2hat"j" - 5hat"k"` in the ratio 3 : 2 is externally.
The position vector of points A and B are `6bar"a" + 2bar"b"` and `bar"a" - 3bar"b"`. If the point C divides AB in the ratio 3 : 2, show that the position vector of C is `3bar"a" - bar"b"`.
Prove that the line segments joining the midpoints of the adjacent sides of a quadrilateral form a parallelogram.
Prove that a quadrilateral is a parallelogram if and only if its diagonals bisect each other.
Find the centroid of tetrahedron with vertices K(5, −7, 0), L(1, 5, 3), M(4, −6, 3), N(6, −4, 2)
If D, E, F are the midpoints of the sides BC, CA, AB of a triangle ABC, prove that `bar"AD" + bar"BE" + bar"CF" = bar0`.
Prove that `(bar"a" xx bar"b").(bar"c" xx bar"d")` =
`|bar"a".bar"c" bar"b".bar"c"|`
`|bar"a".bar"d" bar"b".bar"d"|.`
Prove that the angle bisectors of a triangle are concurrent
If the plane 2x + 3y + 5z = 1 intersects the co-ordinate axes at the points A, B, C, then the centroid of Δ ABC is ______.
If P(2, 2), Q(- 2, 4) and R(3, 4) are the vertices of Δ PQR then the equation of the median through vertex R is ______.
If the position vectors of points A and B are `hati + 8hatj + 4hatk` and `7hati + 2hatj - 8hatk`, then what will be the position vector of the midpoint of AB?
If G and G' are the centroids of the triangles ABC and A'B'C', then `overline("A""A"^') + overline("B""B"^') + overline("C""C"^')` is equal to ______
If the orthocentre and circumcentre of a triangle are (-3, 5, 1) and (6, 2, -2) respectively, then its centroid is ______
If G`(overlineg)` is the centroid, `H(overlineh)` is the orthocentre and P`(overlinep)` is the circumcentre of a triangle and `xoverlinep + yoverlineh + zoverlineg = 0`, then ______
If `3bar"a" + 5bar"b" = 8bar"c"`, then A divides BC in tbe ratio ______.
The co-ordinates of the points which divides line segment joining the point A(2, –6, 8) and B(–1, 3,–4) internally in the ratio 1: 3' are ______.
Find the unit vector in the diret:tion of the vector `veca = hati + hatj + 2hatk`
In ΔABC the mid-point of the sides AB, BC and CA are respectively (l, 0, 0), (0, m, 0) and (0, 0, n). Then, `("AB"^2 + "BC"^2 + "CA"^2)/("l"^2 + "m"^2 + "n"^2)` is equal to ______.
M and N are the mid-points of the diagonals AC and BD respectively of quadrilateral ABCD, then AB + AD + CB + CD is equal to ______.
If `overlinea, overlineb, overlinec` are the position vectors of the points A, B, C respectively and `5overlinea + 3overlineb - 8overlinec = overline0` then find the ratio in which the point C divides the line segment AB.
The position vector of points A and B are `6 bar "a" + 2 bar "b" and bar "a" - 3 bar"b"`. If the point C divided AB in the ratio 3 : 2, show that the position vector of C is `3 bar "a" - bar "b".`
The position vector of points A and B are `6bara +2barb ` and `bara-3barb `.If the point C divides AB in the ratio 3 : 2 then show that the position vector of C is `3bara-barb` .
Find the ratio in which the point C divides segment AB, if `5bara + 4barb - 9barc = bar0`
AB and CD are two chords of a circle intersecting at right angles to each other at P. If R is the centre of the circle, prove that:
`bar(PA) + bar(PB) + bar(PC) + bar(PD) = 2bar(PR)`
If `bara, barb, barc` are the position vectors of the points A, B, C respectively and `5 bar a - 3 bar b - 2 bar c = bar 0`, then find the ratio in which the point C divides the line segment BA.
The position vector of points A and B are `6bara + 2 barb and bara - 3 barb`. If point C divides AB in the ratio 3 : 2, then show that the position vector of C is `3bara - barb`.
The position vector of points A and B are `6bara + 2 barb` and `bara-3 barb`. If the point C divides AB in the ratio 3 : 2 then show that the position vector of C is `3bara -barb`.
The position vector of points A and B are `6bara + 2barb` and `bara - 3barb`. If the point C divides AB in the ratio 3 : 2, then show that the position vector of C is `3bara - barb`.
The position vector of points A and B are 6`bara + 2barb and bara - 3barb`. If the point C divides AB in the ratio 3 : 2 then show that the position vector of C is 3`bara - barb`.
The position vectors of points A and B are 6`bara` + 2`barb` and `bara - 3barb`. If the point C divides AB in the ratio 3:2, then show that the position vector of C is 3`bara - b`.
