Advertisements
Advertisements
प्रश्न
Rahim and Peter go for a morning walk, Rahim walks around a square path of side 50 m and Peter walks around a rectangular path with length 40 m and breadth 30 m. If both of them walk 2 rounds each, who covers more distance and by how much?
उत्तर
Perimeter of the square = (4 × side) units
Side of the square = 50 m
∴ Perimeter = 4 × 50 m = 200 m
Rahim walked twice around the park
∴ Total distance walked by Rahim = 2 × 200 m
Total distance covered by Rahim = 400 m
Perimeter of a rectangle = 2(length + breadth) unit
Length = 40 m
Breadth = 30 m
∴ Perimeter = 2 × (40 + 30) m
= (2 × 70) m
= 140 m
Peter walked around twice
∴ Distance covered by Peter = 2 × 140 m
= 280 m
∴ Distance covered by Peter = 280 m
400 m > 280 m
Difference = 480 – 280
= 120 m
∴ Rahim covers 120 m more than Peter
APPEARS IN
संबंधित प्रश्न
The perimeter of a rectangle is 46 m and its length is 15 m. Find its :
(i) breadth
(ii) area
(iii) diagonal.
The diagonal of a rectangle is 34 cm. If its breadth is 16 cm; find its :
(i) length
(ii) area
The area of a small rectangular plot is 84 m2. If the difference between its length and the breadth is 5 m; find its perimeter.
A rectangular piece of paper has area = 24 cm² and length = 5 cm. Find its perimeter.
The perimeter of a square plot = 360 m. Find:
- its area.
- cost of fencing its boundary at the rate of ₹ 40 per meter.
- cost of levelling the plot at ₹60 per square meter.
Look at the figures on the sheet of graph paper. Measure their sides with the help of the lines on the graph paper. Write the perimeter of each in the right box.

- Perimeter of rectangle ABCD = ______ cm.
- Perimeter of rectangle EFGH = ______ cm.
- Perimeter of square PQRS = ______ cm.
- Perimeter of rectangle STUV = ______ cm.
The length of a rectangle is three times its breadth. If its perimeter is 64 cm, find the sides of the rectangle
How many different rectangles can be made with a 48 cm long string? Find the possible pairs of length and breadth of the rectangles.
In the given figure, perimeter of (ii) is greater than that of (i), but its area is smaller than that of (i).
 |
 |
| (i) | (ii) |
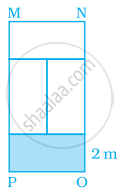
Rectangle MNOP is made up of four congruent rectangles (Figure). If the area of one of the rectangles is 8 m2 and breadth is 2 m, then find the perimeter of MNOP.