Advertisements
Advertisements
प्रश्न
Show that the following sets of points are collinear.
(2, 5), (4, 6) and (8, 8)
उत्तर
The formula for the area ‘A’ encompassed by three points, (x1,y1) (x2,y2)and (x3,y3) is given by the formula,
We know area o triangle formed by three points (x1,y1),(x2,y2),(x3,y3) is given by
`Δ=1/2[x_1(y_2-y_3)+x_2 (y_3-y_1)+x_3(y_1-y_2)] `
If three points are collinear the area encompassed by them is equal to 0
The three given points are A(2, 5), B(4, 6) and C(8, 8). Substituting these values in the earlier mentioned formula we have,
A`=1/2[2(6-8)+4(8-5)+8(5-6)] `
`=1/2[2(-2) +4(3)+8(-1)]`
`=1/2[-4+12-8]`
`=1/2 [-12+12]`
`=0`
Since the area enclosed by the three points is equal to 0, the three points need to be colliner
APPEARS IN
संबंधित प्रश्न
Find the area of the triangle ABC with A(1, −4) and mid-points of sides through A being (2, −1) and (0, −1).
Find the area of the quadrilateral ABCD whose vertices are respectively A(1, 1), B(7, –3), C(12, 2) and D(7, 21).
Show that points A (a, b + c), B (b, c + a), C (c, a + b) are collinear.
Find values of k if area of triangle is 4 square units and vertices are (−2, 0), (0, 4), (0, k)
Prove that the points (2,3), (-4, -6) and (1, 3/2) do not form a triangle.
Prove that the points (a, b), (a1, b1) and (a −a1, b −b1) are collinear if ab1 = a1b.
Find the area of the blades of thc magnetic compass shown in Fig.. 12.27. (Take √11 = 3.32).
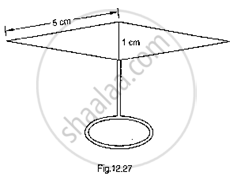
Show that the points A(-5,6), B(3,0) and C(9,8) are the vertices of an isosceles right-angled triangle. Calculate its area.
Show that the following points are collinear:
A(5,1), B(1, -1) and C(11, 4)
The area of a triangle with base 4 cm and height 6 cm is 24 cm2.
