Advertisements
Advertisements
प्रश्न
SN and QM are perpendiculars to the diagonal PR of parallelogram PQRS.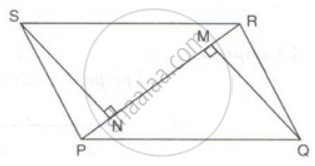
Prove that:
(i) ΔSNR ≅ ΔQMP
(ii) SN = QM
उत्तर
(i) In ΔSNR and ΔQMP
∠SNR = ∠QMP ...(right angles)
∠SRN = ∠MPQ ...(alternate angles since PQ || SR0
∴ ΔSNR ∼ ΔQMP
∠RSN = ∠PQM .........(i)
In ΔSNR and ΔQMP
∠SRN = ∠MPQ
∠RSN = ∠PQM ...(from (i))
PQ = SR ...(PQRS is a parallelogram)
Therefore, ΔSNR ≅ ΔQMP ...(ASA axiom)
(ii) Since ΔSNR ≅ ΔQMP
Hence, SN = QM.
APPEARS IN
संबंधित प्रश्न
PQRS is a parallelogram. T is the mid-point of RS and M is a point on the diagonal PR such that MR = `(1)/(4)"PR"`. TM is joined and extended to cut QR at N. Prove that QN = RN.
P is a point on side KN of a parallelogram KLMN such that KP : PN is 1 : 2. Q is a point on side LM such that LQ : MQ is 2 : 1. Prove that KQMP is a parallelogram.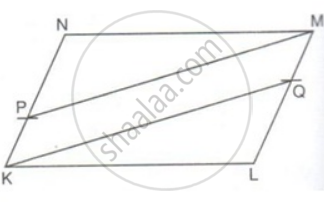
Prove that the line segment joining the mid-points of the diagonals of a trapezium is parallel to each of the parallel sides, and is equal to half the difference of these sides.
In a parallelogram PQRS, M and N are the midpoints of the opposite sides PQ and RS respectively. Prove that
PMRN is a parallelogram.
In the given figure, PQRS is a trapezium in which PQ ‖ SR and PS = QR. Prove that: ∠PSR = ∠QRS and ∠SPQ = ∠RQP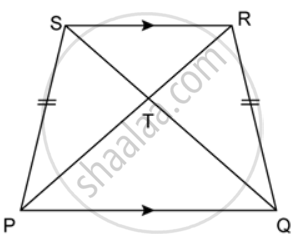
Prove that the diagonals of a kite intersect each other at right angles.
Prove that the diagonals of a parallelogram divide it into four triangles of equal area.
The diagonals AC and BC of a quadrilateral ABCD intersect at O. Prove that if BO = OD, then areas of ΔABC an ΔADC area equal.
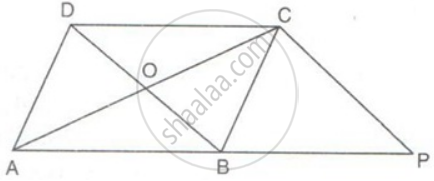
PQRS is a parallelogram and O is any point in its interior. Prove that: area(ΔPOQ) + area(ΔROS) - area(ΔQOR) + area(ΔSOP) = `(1)/(2)`area(|| gm PQRS)
In the figure, ABCD is a parallelogram and CP is parallel to DB. Prove that: Area of OBPC = `(3)/(4)"area of ABCD"`