Advertisements
Advertisements
प्रश्न
The area of a trapezium is 1080 sq.cm. If the lengths of its parallel sides are 55.6 cm and 34.4 cm. Find the distance between them
उत्तर
Length of the parallel sides a = 55.6 cm, b = 34.4 cm
Area of the trapezium = 1080 sq.cm
`1/2 xx "h" xx ("a" + "b")` = 1080
`1/2 xx "h" xx (55.6 + 4.4)` = 1080
`1/2 xx "h" xx 90.0` = 1080
h = `1080/45`
= 24 cm
Distance between parallel sides = 24 cm.
APPEARS IN
संबंधित प्रश्न
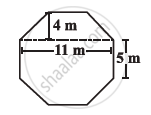
Top surface of a raised platform is in the shape of a regular octagon as shown in the figure. Find the area of the octagonal surface.
Find the area, in square metres, of the trapezium whose bases and altitude is as under:
bases = 28 cm and 3 dm, altitude = 25 cm
Find the area of a trapezium whose parallel sides are of length 16 dm and 22 dm and whose height is 12 dm.
Find the height of a trapezium, the sum of the lengths of whose bases (parallel sides) is 60 cm and whose area is 600 cm2.
Find the area of a trapezium whose parallel sides of lengths 10 cm and 15 cm are at a distance of 6 cm from each other. Calculate this area as the difference of the area of a rectangle and the sum of the areas of two triangles.
The cross-section of a canal is a trapezium in shape. If the canal is 10 m wide at the top 6 m wide at the bottom and the area of cross-section is 72 m2 determine its depth.
The area of a trapezium is 91 cm2 and its height is 7 cm. If one of the parallel sides is longer than the other by 8 cm, find the two parallel sides.
Find the area enclosed by each of the following figures [Fig. 20.49 (i)-(iii)] as the sum of the areas of a rectangle and a trapezium: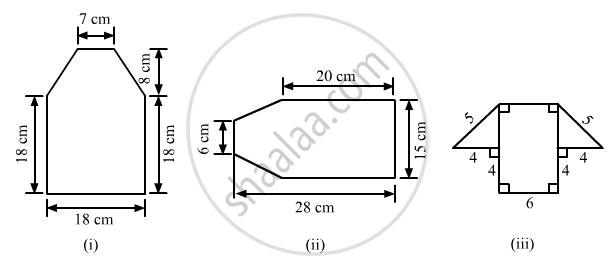
The parallel sides of a trapezium are in ratio 3: 4. If the distance between the parallel sides is 9 dm and its area is 126 dm2; find the lengths of its parallel sides.
The sunshade of a window is in the form of isosceles trapezium whose parallel sides are 81 cm and 64 cm and the distance between them is 6 cm. Find the cost of painting the surface at the rate of ₹ 2 per sq.cm
