Advertisements
Advertisements
प्रश्न
Find the area, in square metres, of the trapezium whose bases and altitude is as under:
bases = 28 cm and 3 dm, altitude = 25 cm
उत्तर
Given:
Bases:
\[28 cm =\frac{28}{100}m = 0.28 m\]
And, 3 dm \[=\frac{3}{10}m = 0.3 m\]
Altitude = 25 cm\[ =\frac{25}{100}m = 0.25 m\]
Area of trapezium \[=\frac{1}{2}\times(\text{ Sum of the bases })\times( \text{ Altitude })\]
\[ = \frac{1}{2} \times (0 . 28 + 0 . 3) m \times (0 . 25) m\]
\[ {= 0.0725 m}^2 \]
APPEARS IN
संबंधित प्रश्न
Find the area of a trapezium whose parallel sides of lengths 10 cm and 15 cm are at a distance of 6 cm from each other. Calculate this area as the difference of the area of a rectangle and the sum of the areas of two triangles.
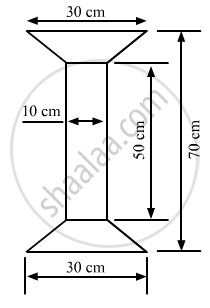
Find the area of Fig. as the sum of the areas of two trapezium and a rectangle.
Mohan wants to buy a trapezium shaped field. Its side along the river is parallel and twice the side along the road. If the area of this field is 10500 m2 and the perpendicular distance between the two parallel sides is 100 m, find the length of the side along the river.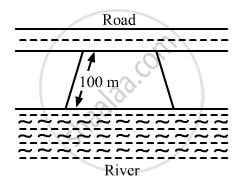
The area of a trapezium is 1586 cm2 and the distance between the parallel sides is 26 cm. If one of the parallel sides is 38 cm, find the other.
Find the area of the field shown in Fig. 20.39 by dividing it into a square, a rectangle and a trapezium.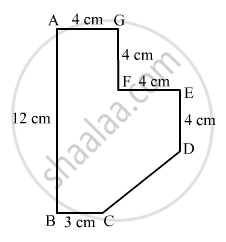
The following figure shows the cross-section ABCD of a swimming pool which is a trapezium in shape.
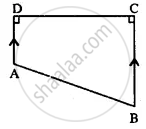
If the width DC, of the swimming pool, is 6.4 m, depth (AD) at the shallow end is 80 cm and depth (BC) at the deepest end is 2.4 m, find its area of the cross-section.
The two parallel sides and the distance between them are in the ratio 3: 4: 2. If the area of the trapezium is 175 cm2, find its height.
In a trapezium if the sum of the parallel sides is 10 cm and the area is 140 sq.cm, then the height is
The area of a trapezium with equal non-parallel sides is 168 m2. If the lengths of the parallel sides are 36 m and 20 m, find the length of the non-parallel sides.
Find the area of the following fields. All dimensions are in metres.

