Advertisements
Advertisements
प्रश्न
The area of the isosceles triangle is `5/4 sqrt(11)` cm2, if the perimeter is 11 cm and the base is 5 cm.
विकल्प
True
False
उत्तर
This statement is True.
Explanation:
Suppose that side of isosceles triangle be a.
Now, perimeter of an isosceles triangle:
2s = 5 + a + a ...[2s = a + b + c]
11 = 5 + 2a
2a = 11 – 5
2a = 6
a = 3
Now, the formula of an area of isosceles triangle = `a/4 sqrt(4b^2 - a^2)`
So, area of an isosceles triangle = `(5sqrt(4 xx (3)^2 - (5)^2))/4`
= `(5sqrt(4 xx 9 - 25))/4`
= `5 xx sqrt(36 - 25)/4`
= `(5sqrt(11))/4` cm2
APPEARS IN
संबंधित प्रश्न
A traffic signal board, indicating ‘SCHOOL AHEAD’, is an equilateral triangle with side ‘a’. Find the area of the signal board, using Heron’s formula. If its perimeter is 180 cm, what will be the area of the signal board?
Find the area of an isosceles triangle having the base x cm and one side y cm.
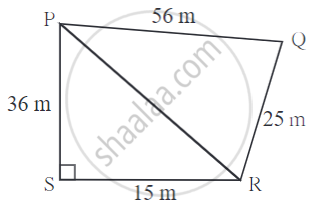
Look at the measures shown in the adjacent figure and find the area of ☐ PQRS.
Find the area of the triangle formed by the points
(–10, –4), (–8, –1) and (–3, –5)
If the points A(– 3, 9), B(a, b) and C(4, – 5) are collinear and if a + b = 1, then find a and b
Find the area of triangle AGF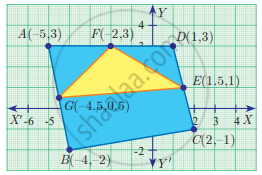
The area of triangle formed by the points (− 5, 0), (0, – 5) and (5, 0) is
Using Heron’s formula, find the area of a triangle whose sides are 1.8 m, 8 m, 8.2 m
The perimeter of an equilateral triangle is 30 cm. The area is
The cost of levelling the ground in the form of a triangle having the sides 51 m, 37 m and 20 m at the rate of Rs 3 per m2 is Rs 918.
