Topics
Number Systems
Number Systems
Polynomials
Algebra
Coordinate Geometry
Linear Equations in Two Variables
Geometry
Coordinate Geometry
Introduction to Euclid’S Geometry
Mensuration
Statistics and Probability
Lines and Angles
- Introduction to Lines and Angles
- Basic Terms and Definitions
- Intersecting Lines and Non-intersecting Lines
- Introduction to Parallel Lines
- Pairs of Angles
- Parallel Lines and a Transversal
- Angle Sum Property of a Triangle
Triangles
- Concept of Triangles
- Congruence of Triangles
- Criteria for Congruence of Triangles
- Properties of a Triangle
- Some More Criteria for Congruence of Triangles
- Inequalities in a Triangle
Quadrilaterals
- Concept of Quadrilaterals
- Properties of a Quadrilateral
- Types of Quadrilaterals
- Another Condition for a Quadrilateral to Be a Parallelogram
- Theorem of Midpoints of Two Sides of a Triangle
- Property: The Opposite Sides of a Parallelogram Are of Equal Length.
- Theorem: A Diagonal of a Parallelogram Divides It into Two Congruent Triangles.
- Theorem : If Each Pair of Opposite Sides of a Quadrilateral is Equal, Then It is a Parallelogram.
- Property: The Opposite Angles of a Parallelogram Are of Equal Measure.
- Theorem: If in a Quadrilateral, Each Pair of Opposite Angles is Equal, Then It is a Parallelogram.
- Property: The diagonals of a parallelogram bisect each other. (at the point of their intersection)
- Theorem : If the Diagonals of a Quadrilateral Bisect Each Other, Then It is a Parallelogram
Circles
Areas - Heron’S Formula
Surface Areas and Volumes
Statistics
Algebraic Expressions
Algebraic Identities
Area
Constructions
- Introduction of Constructions
- Basic Constructions
- Some Constructions of Triangles
Probability
- Collinearity of three points
Notes

Heron was born in about 10AD possibly in Alexandria in Egypt. He worked in applied mathematics. His geometrical works deal largely with problems on mensuration written in three books. In this book, Heron has derived the famous formula for the area of a triangle in terms of its three sides.
The formula given by Heron about the area of a triangle, is also known as Hero’s formula. It is stated as:
| Area of a triangle = `sqrt (s(s-a) (s - b)(s-c))` |
where a, b and c are the sides of the triangle, and s = semi-perimeter, i.e., half the perimeter of the triangle = `(a + b + c) /2`,
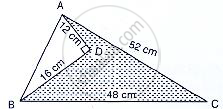
For instances, Let us take a = 40 m, b = 24 m, c = 32 m,
so that we have s = `(40 + 24 + 32 )/ 2` m
= 48 m
s - a = (48 - 40) m = 8m
s - b = (48 - 24)m = 24 m
s - c = (48 - 32 ) m = 16 m
Therefore, area of the park ABC
`= sqrt (s(s - a) (s - b) (s - c))`
`= sqrt (48 * 8 * 24 * 16) m^2 = 384 m^2`