Advertisements
Advertisements
प्रश्न
The base of an isosceles right triangle is 30 cm. Its area is
विकल्प
225 cm2
225 \[\sqrt{3}\] cm2
225 \[\sqrt{2}\] cm2
450 cm2
उत्तर
\[\text{Let ABC be the right triangle in which} \angle B = 90° . \]
\[\text{ Now, base = BC; perpendicular = AB; Hypotenuse = AC } \]
\[\text{ Now, BC = 30 cm } \left( \text{ given } \right)\]
\[\text{ Now, ∆ ABC is an isosceles right angled ∆ and we know that hypotenuse is the longest side of the right ∆ }m. \]
\[\text{ So, AB = BC = 30 cm } \]
\[\text{ area of ∆ ABC } = \frac{1}{2} \times\text{ base } \times \text{ height } \]
\[ = \frac{1}{2} \times BC \times AB\]
\[ = \frac{1}{2} \times 30 \times 30\]
\[ = 450 {cm}^2\]
APPEARS IN
संबंधित प्रश्न
Area of PQRS = Area of PQR + Area of ΔPQS = (6+9.166)𝑐𝑚2=15.166𝑐𝑚2
A park, in the shape of a quadrilateral ABCD, has ∠C = 900, AB = 9 m, BC = 12 m, CD = 5 m and AD = 8 m How much area does it occupy?
Find the perimeter and area of the quadrilateral ABCD in which AB = 17 cm, AD =9 cm, CD = l2cm, ∠ACB = 90° and AC=l5cm.
If each side of a triangle is doubled, the find percentage increase in its area.
The sides of a triangle are 50 cm, 78 cm and 112 cm. The smallest altitude is
The sides of a triangle are 11 cm, 15 cm and 16 cm. The altitude to the largest side is
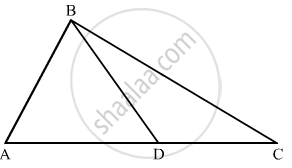
In the given figure, the ratio AD to DC is 3 to 2. If the area of Δ ABC is 40 cm2, what is the area of Δ BDC?
The perimeter of an equilateral triangle is 60 m. The area is ______.
The length of each side of an equilateral triangle having an area of `9sqrt(3)`cm2 is ______.
The sides of a triangle are 35 cm, 54 cm and 61 cm, respectively. The length of its longest altitude ______.
