Advertisements
Advertisements
प्रश्न
The circumcentre of the triangle ABC is O. Prove that ∠OBC + ∠BAC = 90º.
उत्तर
Let ABC be the triangle whose circumcenter is O.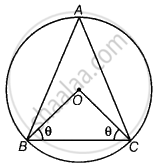
∠OBC = ∠OCB = θ ...(Opposite angles of equal sides)
In ΔBOC, using the angle sum property of tringle, sum of all angles is 180°, we have:
∠BOC + ∠OBC + ∠OCB = 180°
⇒ ∠BOC + θ + θ = 180°
⇒ ∠BOC = 180° – 2θ
Also, in a circle, angle subtended by an arc at the center is twice the angle subtended by it at any other point in the remaining part of the circle.
∠BOC = 2∠BAC
⇒ ∠BAC = `1/2`(∠BOC)
⇒ ∠BAC = `1/2`(180° – 2θ)
⇒ ∠BAC = (90° – θ)
⇒ ∠BAC + θ = 90°
⇒ ∠BAC + ∠OBC = 90°
Hence proved.
APPEARS IN
संबंधित प्रश्न
In the given figure, PQ is a chord of length 8cm of a circle of radius 5cm. The tangents at P and Q intersect at a point T. Find the length TP

Fill in the blanks:
A circle divides the plane, on which it lies, in __________ parts.
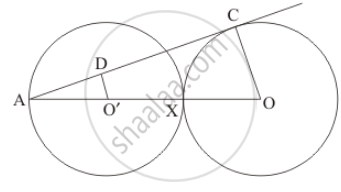
Equal circles with centres O and O' touch each other at X. OO' produced to meet a circle with centre O', at A. AC is a tangent to the circle whose centre is O. O'D is perpendicular to AC. Find the value of\[\frac{DO'}{CO}\]
Radius of a circle with centre O is 4 cm. If l(OP) = 4.2 cm, say where point P will lie.
In following fig. ABC is an equilateral triangle . A circle is drawn with centre A so that ot cuts AB and AC at M and N respectively. Prove that BN = CM.

Mark two points A and B ,4cm a part, Draw a circle passing through B and with A as a center

Draw circle with the radii given below.
4 cm
The longest chord of a circle is __________
In the given figure, if ZRPS = 25°, the value of ZROS is ______

From the figure, identify two points in the interior.

