Advertisements
Advertisements
प्रश्न
If a pair of opposite sides of a cyclic quadrilateral are equal, prove that its diagonals are also equal.
उत्तर
Given: Let ABCD be a cyclic quadrilateral and AD = BC.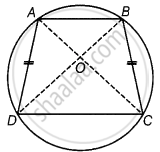
Join AC and BD.
To prove: AC = BD
Proof: In ΔAOD and ΔBOC,
∠OAD = ∠OBC and ∠ODA = ∠OCB ...[Since, same segments subtends equal angle to the circle]
AB = BC ...[Given]
ΔAOD = ΔBOC ...[By ASA congruence rule]
Adding is DOC on both sides, we get
ΔAOD + ΔDOC ≅ ΔBOC + ΔDOC
⇒ ΔADC ≅ ΔBCD
AC = BD ...[By CPCT]
APPEARS IN
संबंधित प्रश्न
If the non-parallel sides of a trapezium are equal, prove that it is cyclic.
Prove that the line of centres of two intersecting circles subtends equal angles at the two points of intersection.
Prove that the circle drawn with any side of a rhombus as diameter passes through the point of intersection of its diagonals.
AC and BD are chords of a circle which bisect each other. Prove that (i) AC and BD are diameters; (ii) ABCD is a rectangle.
In any triangle ABC, if the angle bisector of ∠A and perpendicular bisector of BC intersect, prove that they intersect on the circumcircle of the triangle ABC.
Two chords AB and CD of lengths 5 cm 11cm respectively of a circle are parallel to each other and are on opposite sides of its centre. If the distance between AB and CD is 6 cm, find the radius of the circle.
In the figure, `square`ABCD is a cyclic quadrilateral. Seg AB is a diameter. If ∠ ADC = 120˚, complete the following activity to find measure of ∠ BAC.
`square` ABCD is a cyclic quadrilateral.
∴ ∠ ADC + ∠ ABC = 180°
∴ 120˚ + ∠ ABC = 180°
∴ ∠ ABC = ______
But ∠ ACB = ______ .......(angle in semicircle)
In Δ ABC,
∠ BAC + ∠ ACB + ∠ ABC = 180°
∴ ∠BAC + ______ = 180°
∴ ∠ BAC = ______
Prove that the perpendicular bisectors of the sides of a cyclic quadrilateral are concurrent.
In the given figure, ABCD is a cyclic quadrilateral in which ∠BAD = 75°, ∠ABD = 58° and ∠ADC = 77°, AC and BD intersect at P. Then, find ∠DPC.

In a cyclic quadrilaterals ABCD, ∠A = 4x, ∠C = 2x the value of x is
