Advertisements
Advertisements
प्रश्न
The diameter of the wheel of a car is 70 cm. How many revolutions will it make to travel one kilometer?
उत्तर
The circumference of a circle is given by:
Circumference = π × Diameter.
Here, the diameter of the wheel is 70 cm. Substituting the value of π ≈ 3.1416
Circumference = 3.1416 × 70 = 219.91 cm
Since 1 km = 1000 m = 1000 × 100 = 100,000 cm, the total distance to be traveled is:
1 km = 100,000 cm.
The number of revolutions is the total distance divided by the circumference of the wheel:
Number of revolutions = `("Total distance")/("Circumference") = 100000/219.91`
Number of revolutions ≈ 454.74.
The wheel will make approximately 455 revolutions to travel 1 km.
APPEARS IN
संबंधित प्रश्न
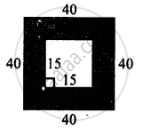
In the figure given below, find the area of shaded region: (All measurements are in cm)
One side of a parallelogram is 20 cm and its distance from the opposite side is 16 cm. Find the area of the parallelogram.
The base of a parallelogram is thrice it height. If its area is 768 cm2, find the base and the height of the parallelogram.
If the area of a rhombus is 112 cm2 and one of its diagonals is 14 cm, find its other diagonal.
The area of a rhombus is 84 cm2 and its perimeter is 56 cm. Find its height.
The area of a right-angled triangle is 160 cm2. If its one leg is 16 cm long, find the length of the other leg.
Find the area of an equilateral triangle whose each side is 16 cm. (Take `sqrt3`= 1.73)
The ratio between the areas of two circles is 16 : 9. Find the ratio between their :
(i) radius
(ii) diameters
(iii) circumference
The diameter of every wheel of a car is 63 cm. How much distance will the car move during the 2000 revolutions of its wheel.
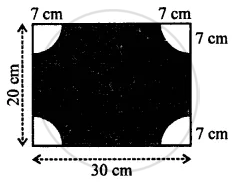
From each corner of a rectangular paper (30 cm x 20 cm) a quadrant of a circle of radius 7 cm is cut. Find the area of the remaining paper i.e., shaded portion.