Advertisements
Advertisements
प्रश्न
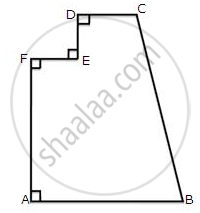
The figure given below shows the cross-section of a concrete structure. Calculate the area of cross-section if AB = 1.8 cm, CD = 0.6 m, DE = 0.8 m, EF = 0.3 m and AF = 1.2 m.
उत्तर
The diagram is redrawn as follows:
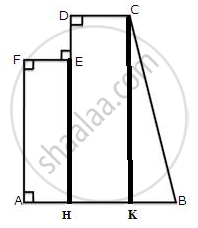
Here
AF = 1.2 m, EF = 0.3m, DC = 0.6m, BK = 1.8 - 0.6 - 0.3 = 0.9 m
Hence,
Area of ABCDEF = Area of AHEF + Area of HKCD + ΔKBC
= 1.2 × 0.3 + 2 × 0.6 + `1/2` × 2 × 0.9
= 0.36 + 2.1
= 2.46 sq.m
APPEARS IN
संबंधित प्रश्न
The diagonal of a quadrilateral shaped field is 24 m and the perpendiculars dropped on it from the remaining opposite vertices are 8 m and 13 m. Find the area of the field.
The distance between parallel sides of a trapezium is 15 cm and the length of the line segment joining the mid-points of its non-parallel sides is 26 cm. Find the area of the trapezium.
The length of a rectangle is twice the side of a square and its width is 6 cm greater than the side of the square. If the area of the rectangle is three times the area of the square; find the dimensions of each.
Calculate the area of quadrilateral ABCD, in which ∠ABD = 90°, triangle BCD is an equilateral triangle of side 24 cm and AD = 26 cm.
Trapezium given below; find its area.
Trapezium given below; find its area.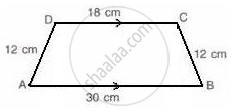
Find the area and perimeter of a square plot of land, the length of whose diagonal is 15 meters. Given your answer correct to 2 places of decimals.
Sum of the areas of two squares is 400 cm2. If the difference of their perimeters is 16 cm, find the sides of the two squares.
Find the area of the quadrilateral whose vertices are at (– 9, 0), (– 8, 6), (– 1, – 2) and (– 6, – 3)
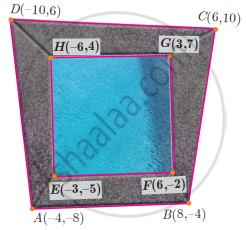
The quadrilateral swimming pool shown is surrounded by concrete patio. Find the area of the patio