Advertisements
Advertisements
प्रश्न
The length of a chain used as the boundary of a semicircular park is 108 m. Find the area of the park.
उत्तर
Let the radius of the park be r.
Length of chain = Perimeter of the semicircular park
⇒ 108 = Length of the arc +Diameter
`=> 108 = 1/2xx2pi"r" + 2"r"`
`⇒ 108 = r (22/7+2) `
`=> 108 = 36/7"r"`
⇒ r = 21 m
Now, Area of park` = 1/2pi"r"^2 = 1/2xx22/7xx(21)^2 = 693 "m"^2`
Hence, the area of the park is 693 m2 .
APPEARS IN
संबंधित प्रश्न
A race track is in the form of a ring whose inner circumference is 352 m, and the outer circumference is 396 m. Find the width of the track.
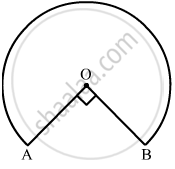
In the given figure, the shape of the top of a table is that of a sector of a circle with centre O and ∠AOB = 90°. If AO = OB = 42 cm,· then find the perimeter of the top of the table.
A copper wire when bent in the form of a square encloses an area of 484 cm2. The same wire is not bent in the form of a circle. Find the area enclosed by the circle.
In the given figure, OPQR is a rhombus, three of whose vertices lie on a circle with centre O. If the area of the rhombus is `32sqrt(3)`, find the radius of the circle.
Find the curved surface area , the total surface area and the volume of a cone if its :
Height = 12 cm , radius = 5 cm
A circus tent is cylindrical to a height of 5 m and conical above it. If its diameter is 42 m and slant height of the cone is 53 m, calculate the total area of the canvas required.
A cylindrical beaker of 7 cm diameter is partly filled with water. Determine the number of spherical marbles of diameter 1.4 cm that are to be submerged in it to raise the water level by 5.6 cm
The diameter of a wheel is 1.4m. How many revolutions does make in moving a distance of 2.2km?
Find the perimeter of the given shape (Take π = `22/7`).
The radius of a circular plot is 7.7 metres. How much will it cost to fence the plot with 3 rounds of wire at the rate of 50 rupees per metre?
