Advertisements
Advertisements
Question
The length of a chain used as the boundary of a semicircular park is 108 m. Find the area of the park.
Solution
Let the radius of the park be r.
Length of chain = Perimeter of the semicircular park
⇒ 108 = Length of the arc +Diameter
`=> 108 = 1/2xx2pi"r" + 2"r"`
`⇒ 108 = r (22/7+2) `
`=> 108 = 36/7"r"`
⇒ r = 21 m
Now, Area of park` = 1/2pi"r"^2 = 1/2xx22/7xx(21)^2 = 693 "m"^2`
Hence, the area of the park is 693 m2 .
APPEARS IN
RELATED QUESTIONS
The inner circumference of a circular track is 220 m. The track is 7m wide everywhere. Calculate the cost of putting up a fence along the outer circle at the rate of j – 2 per metre. (Use π = 22/7)
A sector is cut from a circle of radius 21 cm. The angle of the sector is 150º. Find the length of its arc and area.
The radius of the wheel of a vehicle is 42 cm. How many revolutions will it complete in a 19.8-km-long journey?
The area of a circular ring enclosed between two concentric circles is 88 cm 2. Find the radii of the two circles, if their difference is 1 cm.
The diameter of a right circular cylinder is 12 m and the slant height is 10 m. Find its curved surface area and the total surface area .
Find the volume and the surface area of the spheres in the following :
Radius = 7 cm
Find the total surface area and volume of a hemisphere whose radius is 10 cm.
Construct the circumcircle of the ABC when BC = 6 cm, B = 55° and C = 70°.
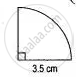
In the adjoining figure, the radius is 3.5 cm. Find:
(i) The area of the quarter of the circle correct to one decimal place.
(ii) The perimeter of the quarter of the circle correct to one decimal place. ( Take π = `22/7`)
A circle with radius 16 cm is cut into four equal parts and rearranged to form another shape as shown in the below figure:

Does the perimeter change? If it does change, by how much does it increase or decrease?
