Advertisements
Advertisements
प्रश्न
The length of the common chord of two intersecting circles is 30 cm. If the diameters of these two circles are 50 cm and 34 cm, calculate the distance between their centers.
उत्तर
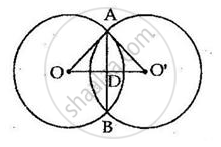
OA = 25 cm and AB = 30 cm
∴ AD = `1/2 xx "AB" = (1/2 xx 30)` cm = 15 cm
Now in right angled ADO
OA2 + AD2 + OD2
⇒ OD2 = OA2 - OD2 = 252 - 152
= 625 - 225 = 400
∴ OD = `sqrt 400` = 20 cm
Again, we have O'A = 17 cm.
In right-angle ADO'
O'A2 = A'D2 + O'D2
⇒ O'D2 = O'A2 - AD2
= 172 - 152
= 289 - 225 = 64
∴ O'D = 8 cm
∴ OO' = ( OD + O'D )
= ( 20 + 8 ) = 28 cm
∴ the distance between their centres is 28 cm.
APPEARS IN
संबंधित प्रश्न
In the following figure, O is the centre of the circle. Find the values of a, b and c.

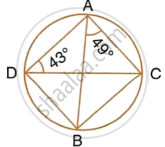
In the following figure, O is the centre of the circle. Find the values of a, b, c and d.
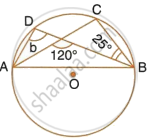
Calculate:
- ∠CDB,
- ∠ABC,
- ∠ACB.
In the figure, chords AE and BC intersect each other at point D. If AD = BD, show that AE = BC.
The line joining the midpoints of two chords of a circle passes through its center.
Prove that the chords are parallel.
Given two equal chords AB and CD of a circle with center O, intersecting each other at point P.
Prove that:
(i) AP = CP
(ii) BP = DP
If a diameter of a circle bisects each of the two chords of a circle, prove that the chords are parallel.
Two chords AB, CD of lengths 16 cm and 30 cm, are parallel. If the distance between AB and CD is 23 cm. Find the radius of the circle.
AB and CD are two chords of a circle such that AB = 6 cm, CD = 12 cm and AB || CD. If the distance between AB and CD is 3 cm, find the radius of the circle.
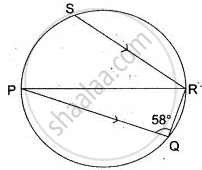
In the adjoining figure, PQ is the diameter, chord SR is parallel to PQ. Give ∠ PQR = 58°.
Calculate:
(i) ∠ RPQ,
(ii) ∠ STP ( T is a point on the minor arc)