Advertisements
Advertisements
प्रश्न
The medians BE and CF of a triangle ABC intersect at G. Prove that the area of ∆GBC = area of the quadrilateral AFGE.
उत्तर
Given: The medians BE and CF of a triangle ABC intersect at G
To prove: ar (ΔGBC) = ar (AFCE)
Proof: As median CF divides a triangle into triangle of equal area. So, ar (ΔBCF) = ar (ΔACF)
ar (ΔGBF) + ar (ΔGBC) = ar (AFGE) + ar (ΔGCE) ...(I)
Now, median BE divides a triangle into two triangle of equal area.
So, ar (ΔGBF) + ar (AFGE) = ar (ΔGCE) + ar (ΔGBC) ...(II)
Now, subtracting (II) from (I), we get
ar (ΔGBC) – ar (AFGE) = ar (ΔAFGE) – ar (ΔGBC)
ar (ΔGBC) + ar (ΔGBC) = ar (ΔAFGE) + ar (ΔAFGE)
2ar (ΔGBC) = 2ar (AFGE)
Hence, ar (ΔGBC) = ar (AFGE).
APPEARS IN
संबंधित प्रश्न
In the given figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that
(i) ar (ACB) = ar (ACF)
(ii) ar (AEDF) = ar (ABCDE)

A villager Itwaari has a plot of land of the shape of a quadrilateral. The Gram Panchayat of the village decided to take over some portion of his plot from one of the corners to construct a Health Centre. Itwaari agrees to the above proposal with the condition that he should be given equal amount of land in lieu of his land adjoining his plot so as to form a triangular plot. Explain how this proposal will be implemented.
ABCD is a trapezium with AB || DC. A line parallel to AC intersects AB at X and BC at Y. Prove that ar (ADX) = ar (ACY).
[Hint: Join CX.]
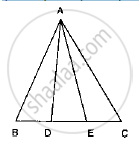
In the below fig. D and E are two points on BC such that BD = DE = EC. Show that ar
(ΔABD) = ar (ΔADE) = ar (ΔAEC).
ABCD is a parallelogram and X is the mid-point of AB. If ar (AXCD) = 24 cm2, then ar (ABC) = 24 cm2.
PQRS is a parallelogram whose area is 180 cm2 and A is any point on the diagonal QS. The area of ∆ASR = 90 cm2.
ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Then ar (BDE) = `1/4` ar (ABC).
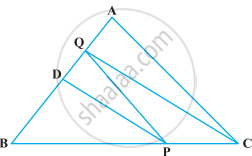
In ∆ABC, D is the mid-point of AB and P is any point on BC. If CQ || PD meets AB in Q (Figure), then prove that ar (BPQ) = `1/2` ar (∆ABC).
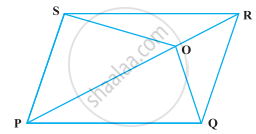
O is any point on the diagonal PR of a parallelogram PQRS (Figure). Prove that ar (PSO) = ar (PQO).
If the medians of a ∆ABC intersect at G, show that ar (AGB) = ar (AGC) = ar (BGC) = `1/3` ar (ABC)
