Advertisements
Chapters
2: Polynomials
3: Coordinate Geometry
4: Linear Equation In Two Variables
5: Introduction To Euclid's Geometry
6: Lines & Angles
7: Triangles
8: Quadrilaterals
▶ 9: Areas of Parallelograms & Triangles
10: Circles
11: Construction
12: Heron's Formula
13: Surface Area & Volumes
14: Statistics & Probability
![NCERT Exemplar solutions for Mathematics [English] Class 9 chapter 9 - Areas of Parallelograms & Triangles NCERT Exemplar solutions for Mathematics [English] Class 9 chapter 9 - Areas of Parallelograms & Triangles - Shaalaa.com](/images/mathematics-english-class-9_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
Advertisements
Solutions for Chapter 9: Areas of Parallelograms & Triangles
Below listed, you can find solutions for Chapter 9 of CBSE NCERT Exemplar for Mathematics [English] Class 9.
NCERT Exemplar solutions for Mathematics [English] Class 9 9 Areas of Parallelograms & Triangles Exercise 9.1 [Pages 85 - 87]
Choose the correct alternative:
The median of a triangle divides it into two ______.
triangles of equal area
congruent triangles
right triangles
isosceles triangles
In which of the following figures, you find two polygons on the same base and between the same parallels?
The figure obtained by joining the mid-points of the adjacent sides of a rectangle of sides 8 cm and 6 cm is ______.
a rhombus of area 24 cm2
a rectangle of area 24 cm2
a square of area 26 cm2
a trapezium of area 14 cm2
In the following figure, the area of parallelogram ABCD is ______.
AB × BM
BC × BN
DC × DL
AD × DL
In the following figure, if parallelogram ABCD and rectangle ABEM are of equal area, then ______.
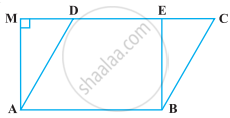
Perimeter of ABCD = Perimeter of ABEM
Perimeter of ABCD < Perimeter of ABEM
Perimeter of ABCD > Perimeter of ABEM
Perimeter of ABCD = `1/2` (Perimeter of ABEM)
The mid-points of the sides of a triangle ABC along with any of the vertices as the fourth point make a parallelogram of area equal to ______.
`1/2` ar (ABC)
- `1/3` ar (ABC)
- `1/4` ar (ABC)
- ar (ABC)
Two parallelograms are on equal bases and between the same parallels. The ratio of their areas is ______.
1 : 2
1 : 1
2 : 1
3 : 1
ABCD is a quadrilateral whose diagonal AC divides it into two parts, equal in area, then ABCD ______.
is a rectangle
is always a rhombus
is a parallelogram
need not be any of (A), (B) or (C)
If a triangle and a parallelogram are on the same base and between same parallels, then the ratio of the area of the triangle to the area of parallelogram is ______.
1 : 3
1 : 2
3 : 1
1 : 4
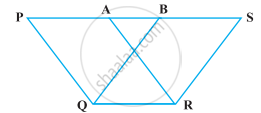
ABCD is a trapezium with parallel sides AB = a cm and DC = b cm (Figure). E and F are the mid-points of the non-parallel sides. The ratio of ar (ABFE) and ar (EFCD) is ______.
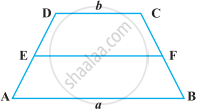
a : b
(3a + b) : (a + 3b)
(a + 3b) : (3a + b)
(2a + b) : (3a + b)
NCERT Exemplar solutions for Mathematics [English] Class 9 9 Areas of Parallelograms & Triangles Exercise 9.2 [Page 88]
State whether the following statement is True or False:
ABCD is a parallelogram and X is the mid-point of AB. If ar (AXCD) = 24 cm2, then ar (ABC) = 24 cm2.
True
False
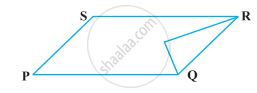
PQRS is a rectangle inscribed in a quadrant of a circle of radius 13 cm. A is any point on PQ. If PS = 5 cm, then ar (PAS) = 30 cm2.
True
False
PQRS is a parallelogram whose area is 180 cm2 and A is any point on the diagonal QS. The area of ∆ASR = 90 cm2.
True
False
ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Then ar (BDE) = `1/4` ar (ABC).
True
False
In the following figure, ABCD and EFGD are two parallelograms and G is the mid-point of CD. Then ar (DPC) = `1/2` ar (EFGD).
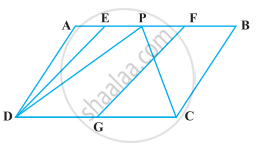
True
False
NCERT Exemplar solutions for Mathematics [English] Class 9 9 Areas of Parallelograms & Triangles Exercise 9.3 [Pages 89 - 92]
In the following figure, PSDA is a parallelogram. Points Q and R are taken on PS such that PQ = QR = RS and PA || QB || RC. Prove that ar (PQE) = ar (CFD).

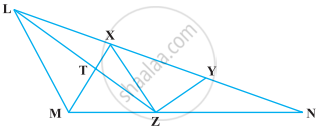
X and Y are points on the side LN of the triangle LMN such that LX = XY = YN. Through X, a line is drawn parallel to LM to meet MN at Z (See figure). Prove that ar (LZY) = ar (MZYX)
The area of the parallelogram ABCD is 90 cm2 (see figure). Find
- ar (ΔABEF)
- ar (ΔABD)
- ar (ΔBEF)
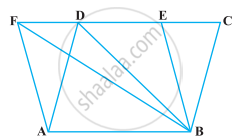
In ∆ABC, D is the mid-point of AB and P is any point on BC. If CQ || PD meets AB in Q (Figure), then prove that ar (BPQ) = `1/2` ar (∆ABC).
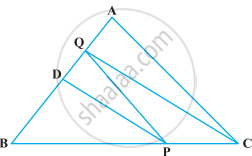
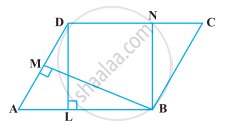
ABCD is a square. E and F are respectively the mid-points of BC and CD. If R is the mid-point of EF (Figure), prove that ar (AER) = ar (AFR)
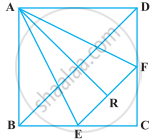
O is any point on the diagonal PR of a parallelogram PQRS (Figure). Prove that ar (PSO) = ar (PQO).
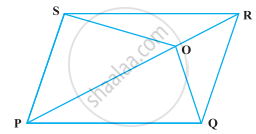
ABCD is a parallelogram in which BC is produced to E such that CE = BC (Figure). AE intersects CD at F. If ar (DFB) = 3 cm2, find the area of the parallelogram ABCD.
In trapezium ABCD, AB || DC and L is the mid-point of BC. Through L, a line PQ || AD has been drawn which meets AB in P and DC produced in Q (Figure). Prove that ar (ABCD) = ar (APQD)
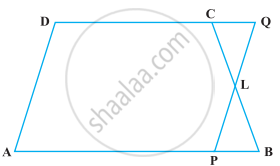
If the mid-points of the sides of a quadrilateral are joined in order, prove that the area of the parallelogram so formed will be half of the area of the given quadrilateral (Figure).
[Hint: Join BD and draw perpendicular from A on BD.]

NCERT Exemplar solutions for Mathematics [English] Class 9 9 Areas of Parallelograms & Triangles Exercise 9.4 [Pages 94 - 96]
A point E is taken on the side BC of a parallelogram ABCD. AE and DC are produced to meet at F. Prove that ar (ADF) = ar (ABFC)
The diagonals of a parallelogram ABCD intersect at a point O. Through O, a line is drawn to intersect AD at P and BC at Q. Show that PQ divides the parallelogram into two parts of equal area.
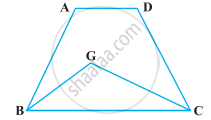
The medians BE and CF of a triangle ABC intersect at G. Prove that the area of ∆GBC = area of the quadrilateral AFGE.
In the following figure, CD || AE and CY || BA. Prove that ar (CBX) = ar (AXY).

ABCD is a trapezium in which AB || DC, DC = 30 cm and AB = 50 cm. If X and Y are, respectively the mid-points of AD and BC, prove that ar (DCYX) = `7/9` ar (XYBA)
In ∆ABC, if L and M are the points on AB and AC, respectively such that LM || BC. Prove that ar (LOB) = ar (MOC)
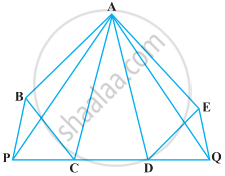
In the following figure, ABCDE is any pentagon. BP drawn parallel to AC meets DC produced at P and EQ drawn parallel to AD meets CD produced at Q. Prove that ar (ABCDE) = ar (APQ)
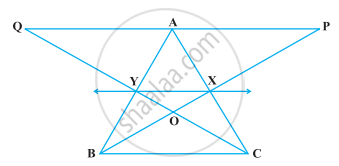
If the medians of a ∆ABC intersect at G, show that ar (AGB) = ar (AGC) = ar (BGC) = `1/3` ar (ABC)
In the following figure, X and Y are the mid-points of AC and AB respectively, QP || BC and CYQ and BXP are straight lines. Prove that ar (ABP) = ar (ACQ).
In the following figure, ABCD and AEFD are two parallelograms. Prove that ar (PEA) = ar (QFD). [Hint: Join PD].

Solutions for 9: Areas of Parallelograms & Triangles
![NCERT Exemplar solutions for Mathematics [English] Class 9 chapter 9 - Areas of Parallelograms & Triangles NCERT Exemplar solutions for Mathematics [English] Class 9 chapter 9 - Areas of Parallelograms & Triangles - Shaalaa.com](/images/mathematics-english-class-9_6:5f2b1b2038084cf381bfa42c826a928c.jpg)
NCERT Exemplar solutions for Mathematics [English] Class 9 chapter 9 - Areas of Parallelograms & Triangles
Shaalaa.com has the CBSE Mathematics Mathematics [English] Class 9 CBSE solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. NCERT Exemplar solutions for Mathematics Mathematics [English] Class 9 CBSE 9 (Areas of Parallelograms & Triangles) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. NCERT Exemplar textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Mathematics [English] Class 9 chapter 9 Areas of Parallelograms & Triangles are Corollary: Triangles on the same base and between the same parallels are equal in area., Corollary: A rectangle and a parallelogram on the same base and between the same parallels are equal in area., Theorem: Parallelograms on the Same Base and Between the Same Parallels., Concept of Area.
Using NCERT Exemplar Mathematics [English] Class 9 solutions Areas of Parallelograms & Triangles exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in NCERT Exemplar Solutions are essential questions that can be asked in the final exam. Maximum CBSE Mathematics [English] Class 9 students prefer NCERT Exemplar Textbook Solutions to score more in exams.
Get the free view of Chapter 9, Areas of Parallelograms & Triangles Mathematics [English] Class 9 additional questions for Mathematics Mathematics [English] Class 9 CBSE, and you can use Shaalaa.com to keep it handy for your exam preparation.