Advertisements
Advertisements
प्रश्न
In the following figure, CD || AE and CY || BA. Prove that ar (CBX) = ar (AXY).

उत्तर
Given: In the following figure, CD || AE and CY || BA
To prove: ar (ΔCBX) = ar (ΔAXY) .
Proof: We know that, triangles on the same base and between the same parallels are equal in areas.
Here, ΔABY and ΔABC both lie on the same base AB and between the same parallels CY and BA.
ar (ΔABY) = ar (ΔABC)
⇒ ar (ABX) + ar (AXY) = ar (ABX) + ar (CBX)
⇒ ar (AXY) = ar (CBX) ...[Eliminating ar (ABX) from both sides]
APPEARS IN
संबंधित प्रश्न
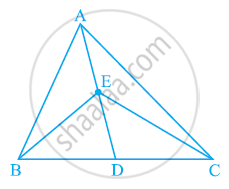
In the given figure, E is any point on median AD of a ΔABC. Show that ar (ABE) = ar (ACE)
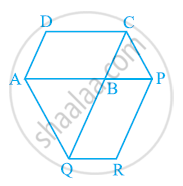
In the given figure, diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD. If AB = CD, then show that:
(i) ar (DOC) = ar (AOB)
(ii) ar (DCB) = ar (ACB)
(iii) DA || CB or ABCD is a parallelogram.
[Hint: From D and B, draw perpendiculars to AC.]

The side AB of a parallelogram ABCD is produced to any point P. A line through A and parallel to CP meets CB produced at Q and then parallelogram PBQR is completed (see the following figure). Show that
ar (ABCD) = ar (PBQR).
[Hint: Join AC and PQ. Now compare area (ACQ) and area (APQ)]
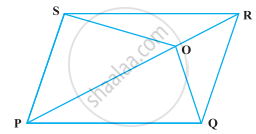
Diagonals AC and BD of a trapezium ABCD with AB || DC intersect each other at O. Prove that ar (AOD) = ar (BOC).
In the given figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that
(i) ar (ACB) = ar (ACF)
(ii) ar (AEDF) = ar (ABCDE)

Diagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD) = ar (BOC). Prove that ABCD is a trapezium.
ABCD is a parallelogram and X is the mid-point of AB. If ar (AXCD) = 24 cm2, then ar (ABC) = 24 cm2.
ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Then ar (BDE) = `1/4` ar (ABC).
O is any point on the diagonal PR of a parallelogram PQRS (Figure). Prove that ar (PSO) = ar (PQO).
In ∆ABC, if L and M are the points on AB and AC, respectively such that LM || BC. Prove that ar (LOB) = ar (MOC)
