Advertisements
Advertisements
प्रश्न
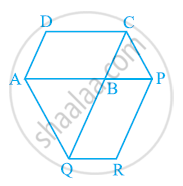
The side AB of a parallelogram ABCD is produced to any point P. A line through A and parallel to CP meets CB produced at Q and then parallelogram PBQR is completed (see the following figure). Show that
ar (ABCD) = ar (PBQR).
[Hint: Join AC and PQ. Now compare area (ACQ) and area (APQ)]
उत्तर
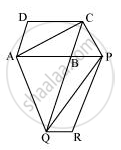
Let us join AC and PQ.
ΔACQ and ΔAQP are on the same base AQ and between the same parallels AQ and CP.
∴ Area (ΔACQ) = Area (ΔAPQ)
⇒ Area (ΔACQ) − Area (ΔABQ) = Area (ΔAPQ) − Area (ΔABQ)
⇒ Area (ΔABC) = Area (ΔQBP) ... (1)
Since AC and PQ are diagonals of parallelograms ABCD and PBQR respectively,
∴ Area (ΔABC) = 1/2Area (ABCD) ... (2)
Area (ΔQBP) = 1/2Area (PBQR) ... (3)
From equations (1), (2), and (3), we obtain
1/2Area (ABCD) = 1/2Area (PBQR)
Area (ABCD) = Area (PBQR)
APPEARS IN
संबंधित प्रश्न
In the given figure, E is any point on median AD of a ΔABC. Show that ar (ABE) = ar (ACE)
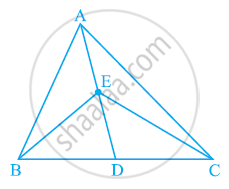
In a triangle ABC, E is the mid-point of median AD. Show that ar (BED) = 1/4ar (ABC).
Show that the diagonals of a parallelogram divide it into four triangles of equal area.
D, E and F are respectively the mid-points of the sides BC, CA and AB of a ΔABC. Show that
(i) BDEF is a parallelogram.
(ii) ar (DEF) = 1/4ar (ABC)
(iii) ar (BDEF) = 1/2ar (ABC)
XY is a line parallel to side BC of a triangle ABC. If BE || AC and CF || AB meet XY at E and F respectively, show that
ar (ABE) = ar (ACF)
In the given figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that
(i) ar (ACB) = ar (ACF)
(ii) ar (AEDF) = ar (ABCDE)

Diagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD) = ar (BOC). Prove that ABCD is a trapezium.
In the given figure, ar (DRC) = ar (DPC) and ar (BDP) = ar (ARC). Show that both the quadrilaterals ABCD and DCPR are trapeziums.
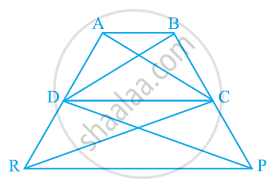
In the following figure, ABCD and EFGD are two parallelograms and G is the mid-point of CD. Then ar (DPC) = `1/2` ar (EFGD).
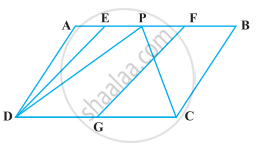
The medians BE and CF of a triangle ABC intersect at G. Prove that the area of ∆GBC = area of the quadrilateral AFGE.
