Advertisements
Advertisements
प्रश्न
Two men on either side of a 75 m high building and in line with base of building observe the angles of elevation of the top of the building as 30° and 60°. Find the distance between the two men. (Use\[\sqrt{3} = 1 . 73\])
उत्तर
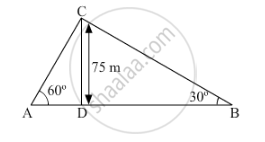
Let CD be the height of the building.
So,
CD = 75 m
It is given that two men are at points A and B and they observe angles of elevation of the top of the building as 60° and 30°, respectively.
\[In ∆ ACD, \]
\[\tan60^o = \frac{CD}{AD}\]
\[ \Rightarrow \sqrt{3} = \frac{75}{AD}\]
\[ \Rightarrow AD = 25\sqrt{3} m\]
\[In ∆ BCD, \]
\[\tan30^o = \frac{CD}{BD}\]
\[ \Rightarrow \frac{1}{\sqrt{3}} = \frac{75}{BD}\]
\[ \Rightarrow BD = 75\sqrt{3} m\]
∴ Distance between both the men = BD + AD =
APPEARS IN
संबंधित प्रश्न
A contractor plans to install two slides for the children to play in a park. For the children below the age of 5 years, she prefers to have a slide whose top is at a height of 1.5 m, and is inclined at an angle of 30° to the ground, where as for the elder children, she wants to have a steep side at a height of 3 m, and inclined at an angle of 60° to the ground. What should be the length of the slide in each case?
A man observes a car from the top of a tower, which is moving towards the tower with a uniform speed. If the angle of depression of the car changes from 30° to 45° in 12 minutes, find the time taken by the car now to reach the tower.
A tree is broken by the wind. The top of that tree struck the ground at an angle of 30° and at a distance of 30. Find the height of the whole tree
From the top of a tower of height 50 m, the angles of depression of the top and bottom of a pole are 30° and 45° respectively. Find
(i) how far the pole is from the bottom of a tower,
(ii) the height of the pole. (Use \[\sqrt{3} = 1 . 732\])
In the following figure, what are the angles of depression from the observing position O1 and O2of the object at A?
When an observer at a distance of 12 m from a tree looks at the top of the tree, the angle of elevation is 60°. What is the height of the tree? `(sqrt 3 = 1.73)`
On a morning walk, three persons step out together and their steps measure 30 cm, 36 cm, and 40 cm respectively. What is the minimum distance each should walk so that each can cover the same distance in complete steps?
The distance of point A(-5, 6) from the origin is ______.
Two towers A and B are standing some distance apart. From the top of tower A, the angle of depression of the foot of tower B is found to be 30°. From the top of tower B, the angle of depression of the foot of tower A is found to be 60°. If the height of tower B is ‘h’ m then the height of tower A in terms of ‘h’ is ____________ m.
A pole of height 30 m is observed from a point. The angle of depression of the point is 30°. Find the distance of the point from the base of the pole.
