Advertisements
Advertisements
प्रश्न
Two right circular cone x and y are made x having three times the radius of y and y having half the volume of x. Calculate the ratio between the heights of x and y.
उत्तर
Let radius of cone y = r
Therefore, radius of cone x = 3r
Let volume of cone y = V
Then volume of cone x = 2V
Let h1 be the height of x and h2 be the height of y.
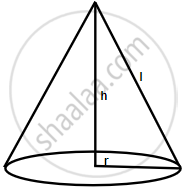
Therefore, Volume of cone =`1/3pir^2h`
Volume of cone `x = 1/3pi(3r)^2h_1`
= `1/3pi9r^2h_1`
= `3pir^2h_1`
Volume of cone `y = 1/3pir^2h_2`
∴ `(2V)/v = (3pir^2h_1)/(1/3pir^2h_2)`
`=> 2/1 = (3h_1 xx 3)/h_2 = (9h_1)/h_2`
`=> h_1/h_2 = 2/1 xx 1/9 = 2/9`
∴ h1 : h2 = 2 : 9
APPEARS IN
संबंधित प्रश्न
A hemispherical bowl of diameter 7.2 cm is filled completely with chocolate sauce. This sauce is poured into an inverted cone of radius 4.8 cm. Find the height of the cone.
The radius of a cone is 5 cm and vertical height is 12 cm. Find the area of the curved surface.
Find the curved surface area of a cone with base radius 5.25 cm and slant height 10cm.
Find the total surface area of a cone, if its slant height is 21 m and diameter of its base is 24 m.
Find the volume of a right circular cone with:
height 21 cm and slant height 28 cm.
The radius and height of a right circular cone are in the ratio 5 : 12 and its volume is 2512 cubic cm. Find the slant height and radius of the cone. (Use it 𝜋 = 3.14).
Find the volume of the largest right circular cone that can be fitted in a cube whose edge is 14 cm.
The curved surface area of a cone is 12320 cm2. If the radius of its base is 56 cm, find its height.
The circumference of the base of a 12 m high conical tent is 66 m. Find the volume of the air contained in it.
The volume of a conical tent is 1232 m3 and the area of the base floor is 154 m2. Calculate the:
- radius of the floor,
- height of the tent,
- length of the canvas required to cover this conical tent if its width is 2 m.
A solid sphere and a solid hemi-sphere have the same total surface area. Find the ratio between their volumes.
From a rectangular solid of metal 42 cm by 30 cm by 20 cm, a conical cavity of diameter 14 cm and depth 24 cm is drilled out. Find :
- the surface area of remaining solid,
- the volume of remaining solid,
- the weight of the material drilled out if it weighs 7 gm per cm3.
Find the height of the cone whose base radius is 5 cm and volume is 75π cm3.
The radius and height of a cylinder, a cone and a sphere are same. Calculate the ratio of their volumes.
The ratio of the base area and the curved surface of a conical tent is 40: 41. If the height is 18 m, Find the air capacity of the tent in terms of n.
A cone and a hemisphere have equal bases and equal volumes. Find the ratio of their heights.
A metallic cylinder has a radius of 3 cm and a height of 5 cm. It is made of metal A. To reduce its weight, a conical hole is drilled in the cylinder, as shown and it is completely filled with a lighter metal B. The conical hole has a radius of `3/2` cm and its depth is `8/9` cm. Calculate the ratio of the volume of the metal A to the volume of metal B in the solid.
The ratio of the radii of two right circular cones of the same height is 1 : 3. Find the ratio of their curved surface area when the height cone is 3 times the radius of the smaller cone.
The curved surface area of a right circular cone of height 15 cm and base diameter 16 cm is
